Since it's isolated (and therefore has no potential energy), the quick answer is that it'sit resembles (but is not exactly) a Gaussian distribution on the order of 10 - 50 angstroms wide. You can calculate it yourself from the equation for thermal wavelength:
$$ \Lambda = \sqrt{\frac{h^2}{2\pi m k_BT}} $$
At room temperature, using the mass of an electron we have $\Lambda = 4.3\times10^{-9}$ meters, or 43 Angstroms.
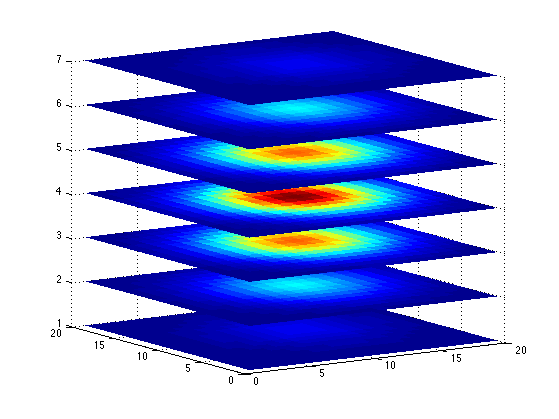
So it would "look" something like this, where red indicates a "denser" part of the "cloud" (though this 2D gaussian doesn't do justice to a 3D function):

This is a 3D gaussian. We have to use cross sections to see the probability density though: