Alan Rominger gave some good information in his answer. Not only some numbers but how to get the numbers. I would like to add to it.
The approach I use is different from Rominger's. I try to explain it here. To get acceleration at elevator foot the model subtracts $\omega^2r$ from $GM_{planet}/r^2$ to get net acceleration. If a moon is the tether anchor, $GM_{moon}/r_{moon}^2$ is also included, whether this acceleration is added or subtracted depends if the tether is above or below the moon.
This net acceleration times kilograms gives the newtons payload exerts. This sets the thickness of the first length of zylon. The next length of tether is thicker as it supports the first length as well as payload. The model chops the tether into a 1000 lengths.
Here is a screen capture from my look at a Mars elevator:
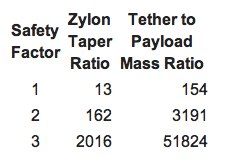
It is reassuring to me that my numbers aren't much different from Rominger's. For a safety factor of one, Rominger gives a Zylon taper ratio of 12.449 (vs 13 for my model). For a safety factor of three he gives a Zylon taper ratio of 1929.560 (vs 2016 for my model).
My model based on Wolfe's spreadsheet also gives ratio of Zylon tether to payload mass. For a safety factor of 1, you'd need 154 times the mass in Zylon as the payload being lifted. By "payload" I mean the elevator car and contents. The elevator car would need its own power source and engines. So the actual cargo would be even less.
With a safety factor of 1, the slightest nick or scrape will cause a break. Given the large length of this elevator, I'd expect breaks to be frequent. I would not risk valuable cargo on such an elevator, much less human lives.
Given a sensible safety factor of three, tether to payload ratio would be 51,824.
And this wouldn't be the entire elevator. There must be tether and a counterweight above Mars synchronous orbit to balance the downward newtons exerted by the lower elevator.
A Zylon Clarke tower for Mars is implausible.
However I don't write off the notion of Mars elevators. Mars equator isn't the only place to anchor an elevator. There are a number of scenarios I look at:
Lower Phobos Elevator
Upper Phobos Elevator
Deimos Elevator
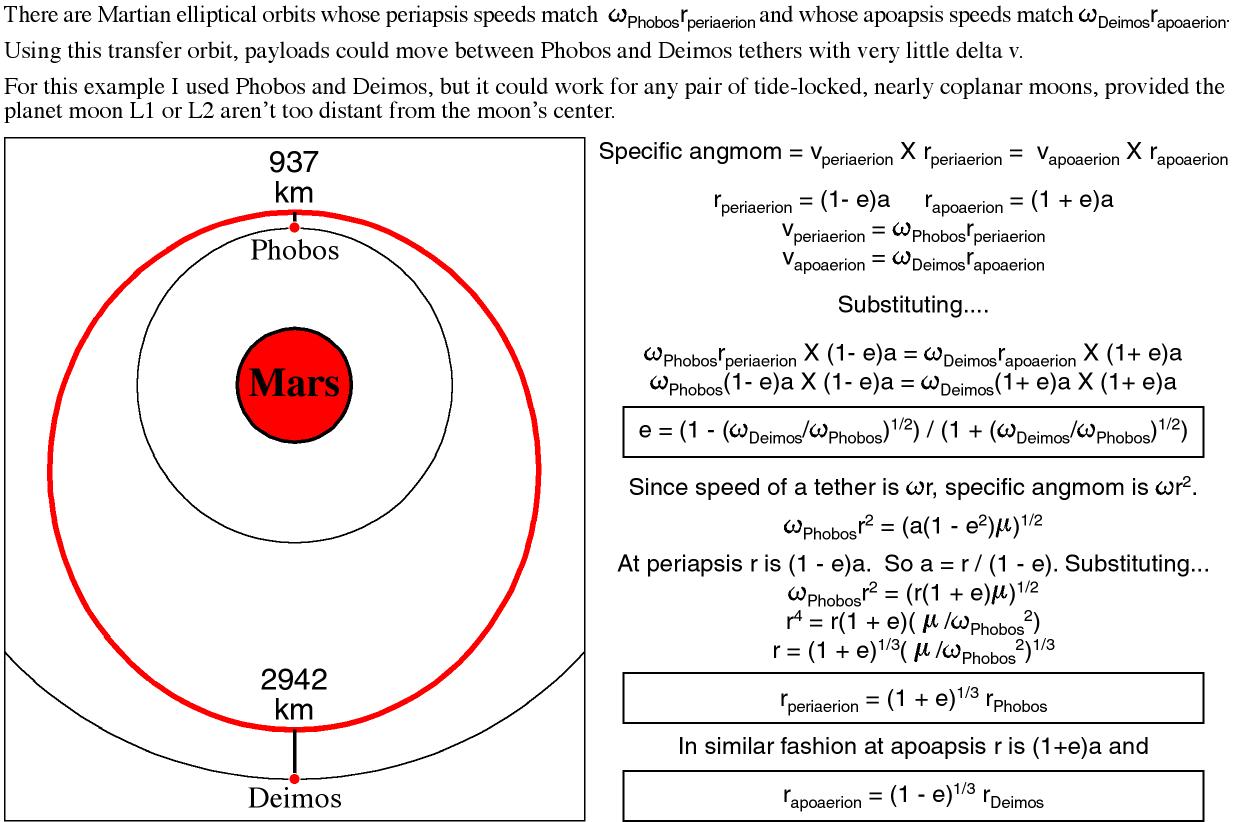
I am excited about elevators allowing a Zero Relative Velocity Transfer Orbit (ZRVTO) between Phobos and Deimos:
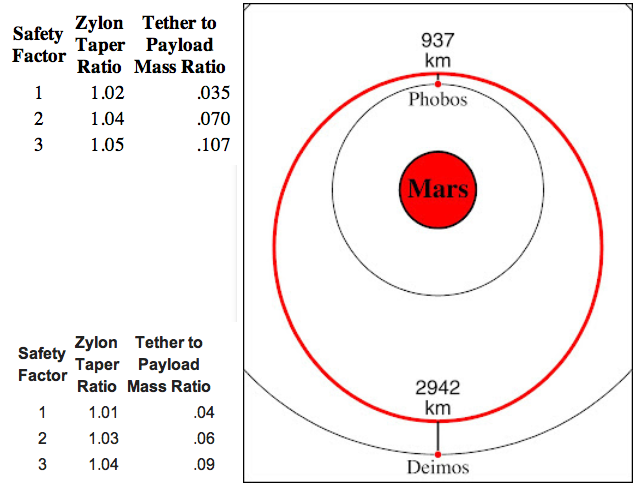
Taper ratios and tether to payload ratios for these two elevators are quite modest:
Extend the upper Phobos elevator and you can fling payloads to the Main Asteroid Belt as well as towards earth. And without prohibitive tether to payload mass ratios.
Extend the lower Phobos elevator 1400 kilometers and you can drop payloads into orbits where periapsis passes through Mars' upper atmosphere. A few periapsis drag passes can circularize at low Mars orbit with a velocity of about 3.4 km/s. EDL would be easier than the 5.5 km/s atmospheric entry a payload incoming from an earth to Mars Hohmann would experience. Especially if the descent vehicle had some propellent and reaction mass from Phobos. Mars EDL could be considerably easier. Given a safety factor of three, tether to payload mass ratio would be .33.
Can a Zylon Phobos elevator descend all the way to Mars upper atmosphere? If so, tether foot velocity with regard to Mars would only be .6 km/s. That's only about mach 2, the Concorde did that. But alas, given a safety factor of three, tether to payload mass ratio would be 638. I don't think a lower Phobos Zylon elevator 5,800 km long is practical.