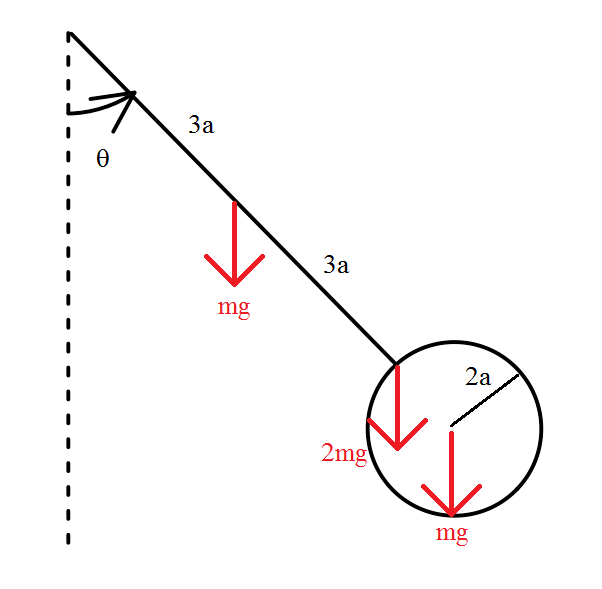
You must be consistent to what you define as positive. Given the picture above, the sum of moments about the pivot point is (considering the positive direction the clockwise direction):
$$ \circlearrowright \sum M=I_o\alpha\\ mg3a\sin \theta+2mg6a\sin \theta+mg8a\sin \theta=(78ma^2+2m(6a)^2)\alpha\\ 23mga\sin \theta=150ma^2\alpha $$$$ \circlearrowright \sum M=I_o\alpha\\ 23mga\sin \theta=150ma^2\alpha $$
But $\theta$ is defined positive counter-clockwise, so $\alpha=-\ddot{\theta}$.
Therefore, the equation of motion
$$ 23mga\sin \theta=-150ma^2\ddot{\theta} $$
The resultant is:
$$ \ddot{\theta} = -\frac{23mga}{150ma^2}\sin \theta=-\frac{23g}{150a}\sin \theta $$$$ \ddot{\theta} = -\frac{23g}{150a}\sin \theta $$
If you define the positive direction the ccw direction, then the LHS of the equation is negative, but $\alpha=\ddot{\theta}$. The final result, however, does not change.