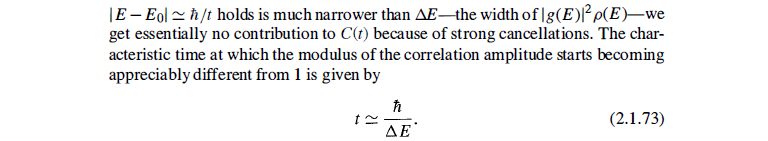The following is a section "Correlation Amplitude and the Energy-Time Uncertainty Relation" from Sakurai's Modern Quantum Mechanics book page 79:
Question:
- What is the importance of the observation that if the interval for which $|E-E_0| \approx \frac{\hbar}{t}$ holds is much narrower than $\Delta E$ then there essentially no contribution to $C(t)$? Lastly, what is essentially the point of these observations?
Thanks.