Suppose we take $100$g of an $x$% by weight solution of sodium chloride, so we have $x$g of salt and $100-x$g of water. The volume of $x$g of salt is:
$$ V_S = x/\rho_S $$
where $\rho_S$ is the density of solid salt. Likewise the volume of the water is:
$$ V_W = (100-x)/\rho_W $$
Suppose when we dissolve salt in water the volumes just add i.e.
$$ V_\text{total} = V_S + V_W = x/\rho_S + (100-x)/\rho_W $$
then the density of our $x$% salt solution would be:
$$ \rho_\text{sol}(x) = \frac{100}{x/\rho_S + (100-x)/\rho_W} \tag{1} $$
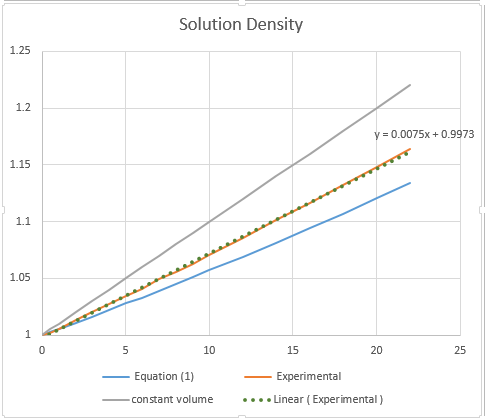
The density of salt is $2.165$g/cm$^3$, and we'll take the density of water to be $1$g/cm$^3$, so we can use equation (1) to calculate what the density would be if the volumes just added and we can compared this with the experimentally measured density. I did this in Excel and got:
$$\begin{matrix} x & Equation (1) & Experimental & Constant Volume\\ 0 & 1.000 & 1.000 & 1\\ 0.5 & 1.003 & 1.002 & 1.005\\ 1 & 1.005 & 1.005 & 1.01\\ 2 & 1.011 & 1.013 & 1.02\\ 3 & 1.016 & 1.020 & 1.03\\ 4 & 1.022 & 1.027 & 1.04\\ 5 & 1.028 & 1.034 & 1.05\\ 6 & 1.033 & 1.041 & 1.06\\ 7 & 1.039 & 1.049 & 1.07\\ 8 & 1.045 & 1.056 & 1.08\\ 9 & 1.051 & 1.063 & 1.09\\ 10 & 1.057 & 1.071 & 1.10\\ 12 & 1.069 & 1.086 & 1.12\\ 14 & 1.081 & 1.101 & 1.14\\ 16 & 1.094 & 1.116 & 1.16\\ 18 & 1.107 & 1.132 & 1.18\\ 20 & 1.121 & 1.148 & 1.20\\ 22 & 1.134 & 1.164 & 1.22 \end{matrix}$$
The experimental densities are greater than the densities calculated using equation (1) i.e. just adding the volumes of the salt and water. That shows the volumes do not simply add but the total volume is less than the sum of the two volumes.