 Source: Electromagnetic Spectrum (Wikipedia)
Source: Electromagnetic Spectrum (Wikipedia)
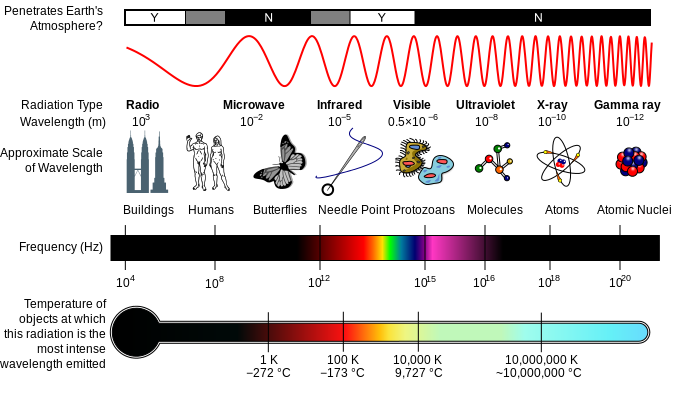
The electromagnetic wave spectrum consists of vaguely defined categories as shown in the picture. Radio waves are a part of it.
In the quantum world, the electromagnetic radiation is carried by a stream of photons. These photons are said to have zero rest mass within the limits of experimental error. A particle having zero rest mass must travel at the speed of light.
We have the following equation from Einstein which holds good in all frames of reference:
$$p = m_ov\gamma = m_ov\frac{1}{\sqrt{1-\frac{v^2}{c^2}}} \tag{1}$$
We also have another equation from Einstein which relates $p$, $m_0$ and $E$:
$$E^2 = (m_oc^2)^2 + (pc)^2 \tag{2}$$
where $m_o$ is the rest mass of the particle, $c$ is the speed of light, $p$ is the momentum of the particle and $v$ is the velocity of the particle.
Since the photon is massless, we get $p$ to be:
$$p = 0 \times \frac{1}{\sqrt{1-\frac{v^2}{c^2}}} \tag{3}$$
If $p$ is zero, then from equation $(2)$, you get $E$ to be zero.
$$E^2 = (0\times c^2)^2 + (0\times c)^2 = 0$$
A particle with zero momentum and zero energy cannot be detected because it does not interact with other particles. For example, if a particle with zero momentum collides with another particle, the particle will not alter the momentum of the other particle if it has to always have zero momentum. We are not interested in undetectable particles for obvious reasons.
So photons don't exist? If you check equation $(3)$ again, you'll notice that if $v = c$, you get a $\frac{0}{0}$. This being indeterminate allows $p$ to take any value (there is one value but we cannot calculate it using equation $(1)$; that's what it means).
We know that photons do have momentum and carry energy. Therefore, we can safely conclude that photons travel at the speed of light ($c$).
If the photons travel at a fixed speed, does that mean that the acceleration of photon must always be zero? Not necessarily. Light can change direction. From general relativity, we know that light gets bent by gravity. By our classical definition of acceleration, light can be told to be accelerated but, however, light is actually moving along the curvature of spacetime, so saying that light is accelerating is not really correct.
Check this questionquestion to get an intuition about light moving in space.
