Why does a simple pendulum or a spring-mass system show simple harmonic motion (SHM) only for small amplitudes?
Simple harmonic motion (in one dimension) is, by definition, a solution to the generic equation $$\frac{d^2x}{dt^2}+\omega^2x=0,$$ where $x$ is a generic variable (it can be for instance a displacement or an angle). This equation of motion can be obtained from Newton's second law $$m\frac{d^2x}{dt^2}=F=-\frac{dU}{dx},$$ where the last equal sign holds for conservative forces, with $U$ being the potential energy.
Therefore we can compare $$\frac{d^2x}{dt^2}+\frac{1}{m}\frac{dU}{dx}=0,$$ with the equation defining the SHM and see that this requires the potential $U$ to be quadratic in $x$.
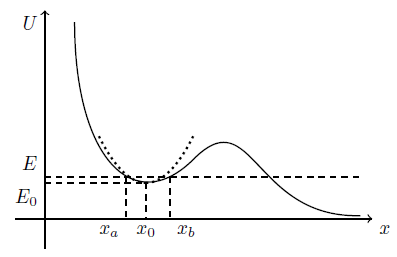
Neither a simple pendulum or a real spring has potential which is quadratic (or parabolic) around an equilibrium point. However in a small enough region around the equilibrium (zero force and minimum of the potential) we can Taylor expand the potential up to second order which just gives a parabolic potential. To understand this, consider the figure below,
This is a generic potential which is definitely not of the required form for a SHM. However, notice asthat we can fit a parabola (dotted line) around any stable equilibrium point such as $x_0$. Around thisIn the vicinity of the equilibrium point $x_0$ we have the potential is parabolicexpansion, $$U(x)=U(x_0)+\frac{dU(x_0)}{dx}(x-x_0)+\frac{1}{2}\frac{d^2U(x_0)}{dx^2}(x-x_0)^2+\mathcal O(\Delta x^3),$$ where $\mathcal O(\Delta x^2)$ means we are neglecting terms of order $(x-x_0)^3$ or greater. Since the first derivative of the potential at $x_0$ gives the force at $x_0$ (which is linearzero), that term vanishes. We are left with $$U(x)=U(x_0)+\frac 12 k(x-x_0)^2++\mathcal O(\Delta x^3),$$ and since $U(x_0)$ is an irrelevant additive term, and restoring$k=frac{d^2U(x_0)}{dx^2}$ is constant, thus the equation of motionthis quadratic potential is exactly the characteristic potential of SHM differential equation. Nearby $x_0$ it gives a linear and restoring force. Note however that this approximation does not hold for arbitrary displacements. At some point $x-x_0$ is so large that higher order terms have to be taken into account.
For a simple pendulum, the potential is $-mgl\cos x$ ($x$ being an angle) whose expansion up two order two around the equilibrium $x=0$ gives a parabola. For a real spring, the potential can be even more complicated, in general it is a sum of terms $a_nx^{2n}$. Then for a small region we can drop higher order terms and keep only the leading one $a_1x^2$ which gives the Hook Law.