Theory
An electromagnetic radiation is monochromatic if all constituents of this radiation will have the same wavelength respectively the same frequency respectively the same energy content. An examples for EM radiation is a light bulb. This light is hardly monochromatic because the electrons which emit the photons are excited on different levels.
Somehow an exception are sodium-vapor lamps, for which Wikipedia says:
These lamps produce a virtually monochromatic light averaging a 589.3 nm wavelength (actually two dominant spectral lines very close together at 589.0 and 589.6 nm).
What about a laser? Here a picture from Wikipedia about Laser:
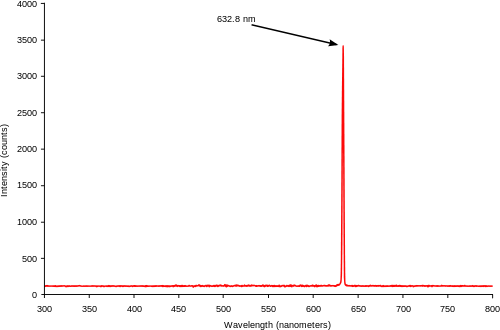
Spectrum of a helium neon laser illustrating its very high spectral purity (limited by the measuring apparatus). The 0.002 nm bandwidth of the lasing medium is well over 10,000 times narrower than the spectral width of a light-emitting diode.
Taking in account that the engineering possibilities to create pure crystals and constant temperatures and constant currents and so on are limited then an bandwidth of 2 picometre are nearly monochromatic.
An electromagnetic pulse is an electromagnetic radiation of a certain duration. To say it with Wikipedia:
A rapid, transient change in the amplitude of a signal from a baseline value to a higher or lower value, followed by a rapid return to the baseline value.
So switching on and off a light bulb we create a pulse. But if this light is not monochromatic, the pulse then more is not monochromatic. The emitting photons electrons will emit at different temperatures and by this emit photons of more different energy values than in the continuous mode.
The same dilemma for a laser pulse. Switching on and off the power source the lasers crystal heatsupheats up and cools down and the bandwith will be broader.
Practice
Which possibilties do we have to create a monochromatic pulse? @PhysicsDave mentiones in his answer a
.. small diamond crystal to generate single photons of light.
- Suppose that the pulses for the stimulation of the photon emissions are following one by one at a constant frequency (this is important because each pulse increases the thermal energy of the crystal) and suppose that the heat dissipation is equilibration with the heating from the pulses. So suppose we are able to stabilize the thermal energy of the crystal at a constant level.
- Suppose the phonon oscillations of the crystal - stimulated from the external pulses - are a whole multiplicity of this pulses.
- Suppose futhermore that the stimulating pulses are hitting always the same atoms respectively the electrons on the same places in the crystal structure.
Even if all supposes would be solvable, the last one is not solvable under rommroom temperature conditions. The electrons are dislocated and this dislocation has a random distribution. Never under such conditions theoretical one will get monochromatic photons. Would it be possible to create photons which are "more" monochromatic as the detection methods I don't know.
Cooling a material nearly to zero and using material, in which the atoms have integer spins (like helium-4) this atoms wil behave as an particle and a excitision should lead to a photons emission at one wavelength. Unfortunately - if i remember right - only some part of the helium-4 atoms are in superposition, so even for superfluid helium-4 one will not get only one wavelength.
