The simple answer is "look at the heat capacity".
For a simple mono-atomic gas, the heat capacity is $\frac32 R$ per mole. For a diatomic gas, it's $\frac52 R$ - because two new modes (rotations) appear that can be excited and contain energy ... after which the equipartition principle does the rest.
Note that the diatomic gas does not have a heat capacity of $\frac72 R$ as you would expect if rotation about its axis or vibration along its axis were involved in storing energy. The reason is that these modes are quantized - and that the thermal energies are insufficient to excite a reasonable fraction of the molecules into the higher energy state. Thus the assumptions that go into the equipartition principle don't apply - and the heat capacity reflects that.
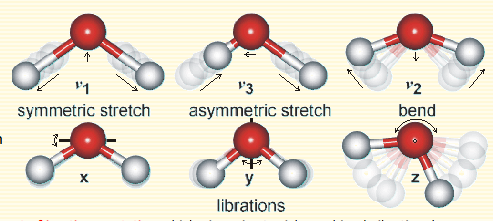
If you have more complex molecules, you can get certain bending modes that will be excited more easily - these are capable of acting as reservoirs of thermal energy, and will affect the heat capacity. For such gases the answer would be "yes".
Note that for water, a significant additional complication is the formation of hydrogen bonds between molecules at lower temperatures; this affects the heat capacity of water in the liquid form in particular (heat capacity changes with temperature for liquid water; and to a lesser degree, for steam). According to this table, the heat capacity of steam actually increases with temperature. This suggests that the vibration modes become more important as temperature goes up. This is even more evident in the heat capacity for liquid water: while it is initially almost flat at 4.2 kJ/Kg/K, around 260°C it rises to 5 kJ/kg/K and then quickly to 10 kJ/kg/K around 250 °C.
There is a good write-up of all this on wikipedia - where it is stated that the energy spacing of the vibrational modes is inversely proportional to the reduced mass of the molecule: this implies that "heavy" diatomic molecules (like $\rm{Br_2}$) have their vibrational mode excited, while the lighter ones do not. An idealized plot of the heat capacity of hydrogen as a function of temperature (from http://theory.physics.manchester.ac.uk/~judith/stat_therm/node81.html which credits P. Eyland from the University of New South Wales ... but I could not locate the original) looks like this:
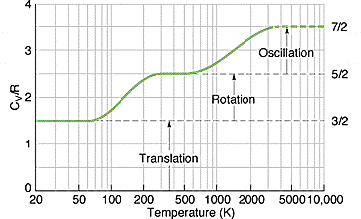
showing that the heat capacity increases first when the rotational modes are accessible for equipartition of energy, and that vibrational modes follow at some (much) higher temperature.