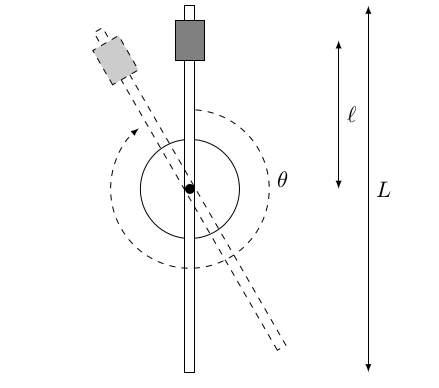
There is a pendulum as shown in the image, with a disc, a rod, and a weight on the rod, pivoted at the rod's center. The masses of the rod and weight are $M$ and $m$, respectively; the mass of the disc is unknown. $L$ is the length of the rod and $\ell$ is the distance from the center to the center of mass of the weight. As the pendulum is released from the vertical position, it swings and comes up short at an angle of $\theta$ as pictured due to a frictional torque.
My questions are:
Is it correct to say the work done by the frictional torque is $mg\ell(1 - \cos\theta)$? (This is the loss in potential energy... but then what is the difference between this and the work done by the torque from gravity?) If not, could you please give me a hint?
Is it correct to write $$I\alpha = \tau_n + \tau_g$$ where $I$ is the total moment of inertia, $\alpha$ is the angular acceleration, $\tau_n$ is the frictional torque, and $\tau_g$ is the torque from gravity? If yes, is it correct to integrate both sides over angular position so that I can find $I$? (I know $\alpha$ as a function of $\theta$the angular position.) Could you please give me a hint if I am wrong?