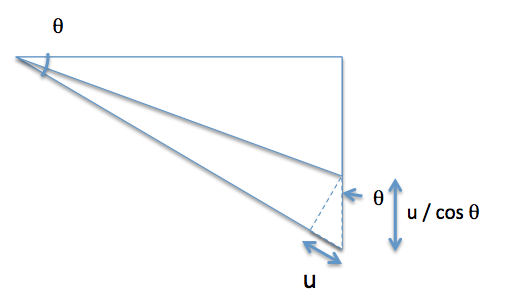
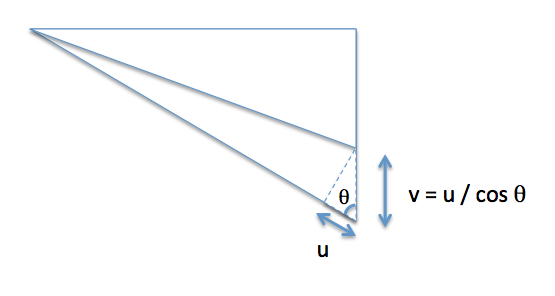
I believe this diagram shows clearly how the approach of your first student can be explained:
From similar triangles, you can see that when the rope gets shorter by distance $u$, the load moves vertically by a distance $\frac{u}{\cos\theta}$.
As for the fallacy of the second student's approach: while velocities are vectors, and vectors can be summed, summation only makes sense when you are considering motion in different frames of reference. If I am in a train moving at velocity $\vec v$, and I throw a ball out of the window at velocity $\vec u$, a person on the ground would see the ball moving at $\vec v + \vec u$. But when two people on the train see the same ball moving at $u$, you can't say "well, A saw a velocity of $u$, and B saw a velocity of $u$, so the object is moving at $2u$"...