This is a very broad subject, but as a rule of thumb, highly non-linear means that the non-linearities cannot be treated with perturbation theoryperturbation theory, as these are not negligible as compared to the linear part of the equations (and, in general, they not only are non-negligible, but actually dominate the dynamics).
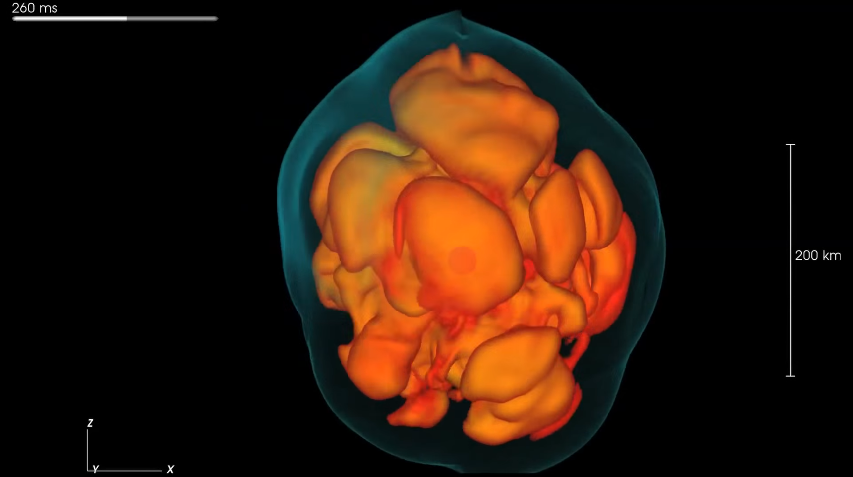
As an example of a non-linear theory which can be treated in perturbation theory, consider QED. On the other hand, highly non-linear equations can be anything that models turbulenceturbulence, specially in the case of general relativity: e.g., the modelisation of the dynamics of a supernova (watch. For a very clear example of a highly non-linear system, I recommend you to watch this simulation of the colapsecollapse of a core: Now Playing: Core Collapse).: