It looks like those patches consist of wave patterns of much shorter wavelength than the rest of the river/lake. This could be an effect of capillary waves which are driven by wind/rain, while the oscillation itself is sustained by capillary forces.
Water wave theory conceptualizes waves as either being governed by gravity or capillary forces. Le Mehaute, "An introduction to hydrodynamics and water waves" is a wonderful book that explains those concepts with passion and detail. Gravity waves follow the dispersion relation $$ w(k) = \sqrt{gk\cdot\tanh{kd}} $$ with water depth $d$, angular frequency $\omega$ and wave number $k\propto1/L$. The interesting point here is that the wave's celerity (velocity) $c=\omega/k$ depends on water depth, $c\propto\sqrt{d}$ only under shallow conditions, $d/L\to0$ (because $\tanh{x}=x$ for $x\to0$). While it does not under deep-water conditions, $c\propto\sqrt{L}$ for $d\to\infty$. Wave crests at the beach are the best illustration for this effect, as the deep-water waves are approaching shallow terrain and thus get slowed down, while their crest (at higher $d$) is still fast.
Note also that shallow-water waves are non-dispersive (celerity does not depend on wavelength), which could explain a longer persistent wave pattern compared to deep water waves.
In contrast, capillary waves are only driven by surface curvature effects. Their short wavelengths justify the deep-water approximation $d\gg L$ in almost any water body, which leads to the dispersion relation $$ \omega(k) = \sqrt{k^3\sigma/\rho} $$
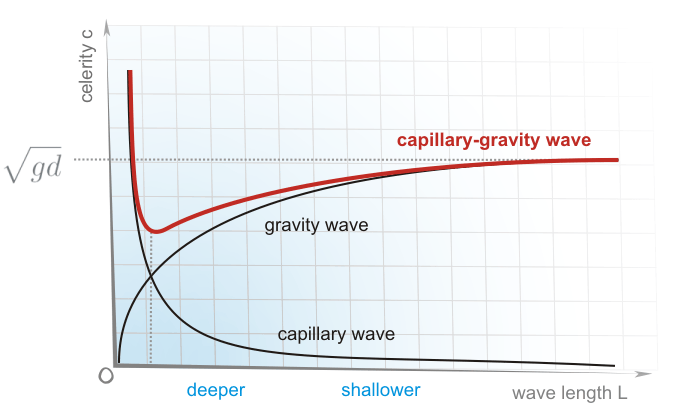
Their combined behaviour can be described as a dispersion relation for capillary-gravity waves, which is illustrated in the following sketch:
This curve shows a critical wavelength $L_\text{crit}\approx 1.7$ cm and a minimum celerity (speed) $c_\text{min}=\sqrt[4]{4g\frac{\sigma}{\rho}}\approx 23$ cm/s for water of typical surface tension $\sigma=0.073$ N/m and density $\rho=1025$ kg/m$^3$.
I would hypothesize that the patches that you see consist of waves oscillating around the critical wave length of 1.7 cm and below, where capillary forces determine the nice looking short-wave pattern. Consequently, this part of the river can be interpreted as deep water, while smoother/longer waves indicate non-dispersive low-velocity waves, $c\propto\sqrt{d}$, in shallow water.
IIt seems that this approach could make sense with respect to the patterns in your images and the likely topography below the surface. But also under-water plants and vegetation will certainly have an effect, though.