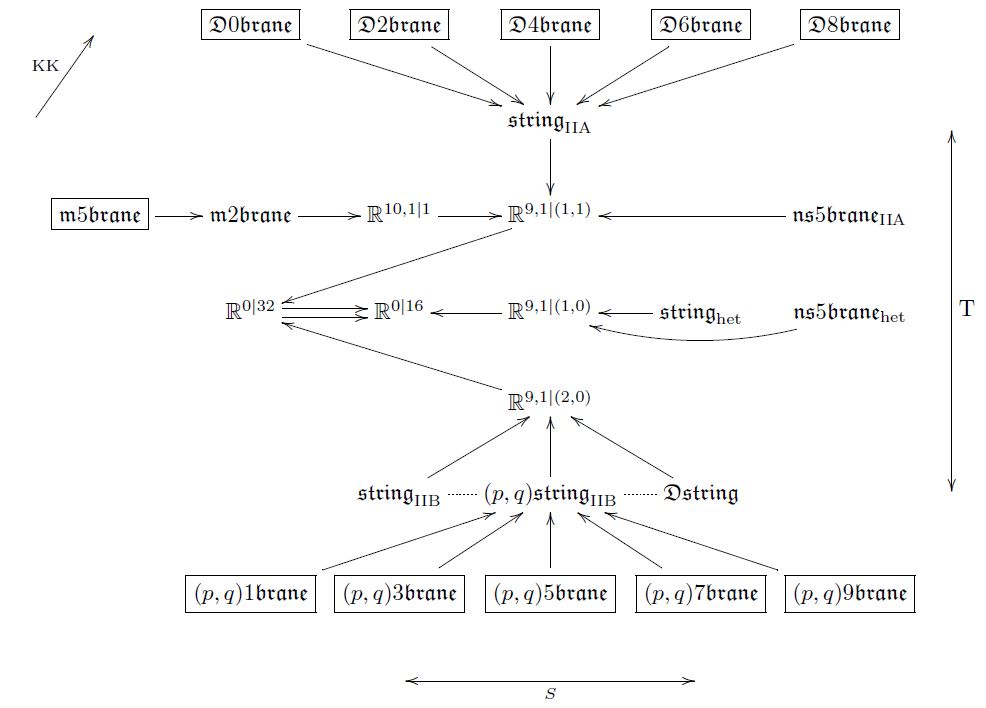
The systematic way to deduce the brane content of string/M-theory is to classify the WZW-terms ($\kappa$-symmetry terms) for the would-be [Green-Schwarz action functionals][2]Green-Schwarz action functionals for the possible super $p$-branes (the "brane scan" or "[brane bouquet][3]""brane bouquet").
Doing this for the would-be D-branes for the heterotic string, one proves that the required non-trivial cocycles don't exist.
This is shown in
C. Chryssomalakos, José de Azcárraga, J. M. Izquierdo and C. Pérez Bueno, "The geometry of branes and extended superspaces", Nuclear Physics B Volume 567, Issues 1–2, 14 February 2000, Pages 293–330 ([arXiv:hep-th/9904137][4]arXiv:hep-th/9904137)
see footnote 14 there, a side remark in the corresponding computation which finds the D-branes in type IIA this way.
The analogous computation classifying the type IIB branes is in section 2 of
Makoto Sakaguchi, "IIB-Branes and New Spacetime Superalgebras", JHEP 0004 (2000) 019 (arXiv:hep-th/9909143).
One may organize these computations in a classifying "bouquet" of super Lie $n$-algebra extensions, which shows the brane spectrum in string/M-theory systematically from super Lie $n$-algebra cohomology:
Here each item denotes a super Lie $n$-algebra and each edge denotes a Lie $n$-algebra extension classified by the relevant super Lie $n$-algebra cocycle needed for the Green-Schwarz sigma-model. Moreover, for every edge the brane species that it points to may end on the brane species that it starts at.
The un-boxed items are those branes appearing already in the "old brane scan", while the boxed items are those branes appearing only as one generalized from super Lie algebras to super Lie $n$-algebras. Since there are no relevant extensions over the super Lie 2-algebra coresponding to the heterotic string, there are no branes that it may end on, in contrast to the type II strings.
For more see
Domenico Fiorenza, Hisham Sati, Urs Schreiber, "[Super Lie $n$-algebra extensions, higher WZW models and super $p$-branes with tensor multiplet fields][5]""Super Lie $n$-algebra extensions, higher WZW models and super $p$-branes with tensor multiplet fields", International Journal of Geometric Methods in Modern Physics, Volume 12, Issue 02 (2015) 1550018 ([arXiv:1308.5264][6]arXiv:1308.5264) [1]: https://i.sstatic.net/C8Pzr.jpg [2]: https://ncatlab.org/nlab/show/Green-Schwarz%20action%20functional [3]: https://ncatlab.org/schreiber/show/The%20brane%20bouquet [4]: https://arxiv.org/abs/hep-th/9904137 [5]: https://ncatlab.org/schreiber/show/The%20brane%20bouquet [6]: https://arxiv.org/abs/1308.5264