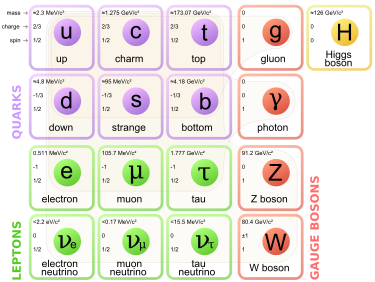
This is the elementary particle table of the standard model of particle physics.
Please note that it is not only the muon that decays, but also the tau and the Z and, W and Higgs.
They are called elementary because they are the building blocks of the Standard Model; building up all other particles and controlling interactions in the microworld where quantum mechanics is necessary to calculate and predict the behavior of particles, using the Standard Model.
Decays are not a unique indication of the existence of a substructure. The substructure is investigated in scattering experiments fitted with the standard model functions. The elementary particles in the table are called point particles because they have no substructure as a hypothesis of the model, and the model is continuously validated, i.e. it has not been falsified.As the diagram provided by @Statics shows, there is a point vertex in the weak decay of the muon ( as also of the tau and the Z and the W). All particles in the table are considered point particles when they interact. No space for constituents.
String theories aim to extend the standard model, describe elementary particles as strings, but this is a subject of research, and still there are no constituents in the string representation of a particle.
Addition after more recall.
How was the compositeness of the nucleus of the atoms established? By the famous Rutherford experiment which showed deep inelastic scattering.
The same scattering experiments at higher energies showed deep inelastic scattering in the protons, establishing the existence of quarks and gluons.
In general scattering experiments established the Form factors of the targets, the "shape" in space and energy/momentum space of the targets, see figure 11 here. That the proton and neutron were not point particles was established long before the discovery of the quark content, and Feynman had proposed his parton model to model the data. Experiments showed deviations from the parton model which established the hard scattering on scattering centers by the so called high p_t data.
No such structure has appeared for the electron within the energy range of our experiments, its size point like within 10^-18 meters within $10^{-18}$ meters, and its shape spherical to great accuracy ..
The symmetries in the standard model of physics are then utilized to posit a point like structure for the elementary particles in the table. At the moment the success of the standard model in describing the experimental data does not leave space for compositeness of elementary particles. If it exists, much higher energies have to be reached in our experiments to discover it.