As far as in general terms how we measure the curvature of space, we can use geometrical methods, rather than units of any particular coordinate system, to establish the deviation of curved space compared to flat space. Such a curvature measurement is based around a unitless ratio.
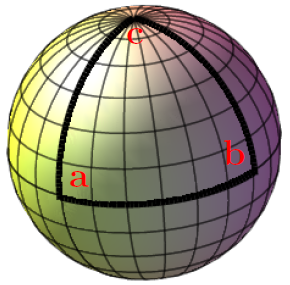
Positive curvature will result in a triangle having a total internal angle of greater than 180 degrees, and negative curvature produces a triangle of total internal angle of less than 180 degrees. More on how we can estimate intrinsic curvature on various toplogical surfaces can be found at Gaussian Curvature, Gaussian curvature is defined not as the angular deficit but as the ratio of the angular deficit to the area of the triangle.
The fundamental difference between intrinsic curvature and extrinsic curvature is that to calculate intrinsic curvature, we do not need an extra dimension (which we don't have available to us in 4 dimensional spacetime).
From left to right: a surface of negative Gaussian curvature (hyperboloid), a surface of zero Gaussian curvature (cylinder), and a surface of positive Gaussian curvature (sphere).
Positive curvature of 270 degrees, rather than as in flatspace, the usual 180 degrees of a triangle.
I am deliberately going to skip over the concept of Parallel Transport and go straight to the Geodetic Effect.
In a curved three-dimensional space, a gryoscope is a good physical object analog for a three-dimensional tangent vector.
A gyroscope in orbit around Earth will point in a given direction, and due to Earth's curvature of spacetime, the direction it points will rotate due to the curvature of spacetime caused by the mass of the Earth. This rotation is called the geodetic effect, and the illustration below exaggerates this effect, as it is not detectable by sight, due to the relatively small mass of the Earth.
This gyroscope based method does actually produce a numerical, rather than geometrical, measurement.
An exaggerated representation of the geodetic effect. A gyroscope placed in orbit about the earth precesses due to the curvature of space around the Earth.
There are other effects, such as Frame Dragging Wikipedia , and a good source of more information, from which the above summary and illustrations are taken is The Geodetic Effect.
I also include a comment from Jerry Schirmer: I'd argue that Riemann curvature definitely has a unit -- inverse length squared. Note that the deviation of triangles from 180 degrees depends on the size of the triangle.