The parton model assumes that nucleons are composed of three valence quarks that share the momentum of the nucleon in an approximately equitative way. That means that the valence quark pdf's have a peak around $x = 1/3$, and have much lower values at $x \to 0$ and $x \to 1$.
In addition to that, you have interactions between quarks (nowadays we know that these interactions are described by QCD, but the details are not too important). The interactions produce loops of virtual quark/antiquark pairs, which constitute the quark sea. Virtual particles with low energy, and therefore low momentum, are easier to produce than energetic virtual particles (in fact, if the quarks are massless, there is an infrared divergence). In conclusion, the quark sea is dominated by quarks with $x=0$, and in the limit $x\to 0$, $S(x) \gg u_v(x), d_v(x)$$S(x) \gg u_V(x), d_V(x)$. The ratio of the proton and neutron form-factors is $$\lim_{x\to 0}\frac{F_2^{en}(x)}{F_2^{ep}(x)} = \lim_{x\to 0} \frac{4 d_v(x) + u_v(x) + 10 S(x)}{4 u_v(x) + d_v(x) + 10S(x)} = \frac{10S(0)}{10S(0)} = 1$$$$\lim_{x\to 0}\frac{F_2^{en}(x)}{F_2^{ep}(x)} = \lim_{x\to 0} \frac{4 d_V(x) + u_V(x) + 10 S(x)}{4 u_V(x) + d_V(x) + 10S(x)} = \frac{10S(0)}{10S(0)} = 1$$
On the other hand, at $x\to 1$ is very difficult to produce energetic virtual particles, so $S(x) \ll u_v(x), d_v(x)$$S(x) \ll u_V(x), d_V(x)$. Here, the difference of masses (which breaks the isospin symmetry) becomes important, since light particles gain more momentum in scattering processes than the heavier ones. The lightest quark is the up quark, so we expect that $u_v(x) \gg d_v(x)$$u_V(x) \gg d_V(x)$, and the ratio of form factor is now $$\lim_{x\to 1}\frac{F_2^{en}(x)}{F_2^{ep}(x)} = \lim_{x\to 1} \frac{4 d_v(x) + u_v(x) + 10 S(x)}{4 u_v(x) + d_v(x) + 10S(x)} = \frac{u_v(1)}{4u_v(1)} = \frac{1}{4} $$$$\lim_{x\to 1}\frac{F_2^{en}(x)}{F_2^{ep}(x)} = \lim_{x\to 1} \frac{4 d_V(x) + u_V(x) + 10 S(x)}{4 u_V(x) + d_V(x) + 10S(x)} = \frac{u_V(1)}{4u_V(1)} = \frac{1}{4} $$
You can compare this approximations with the predictions of some models (lines, triangles) and empirical results (squares, diamonds):
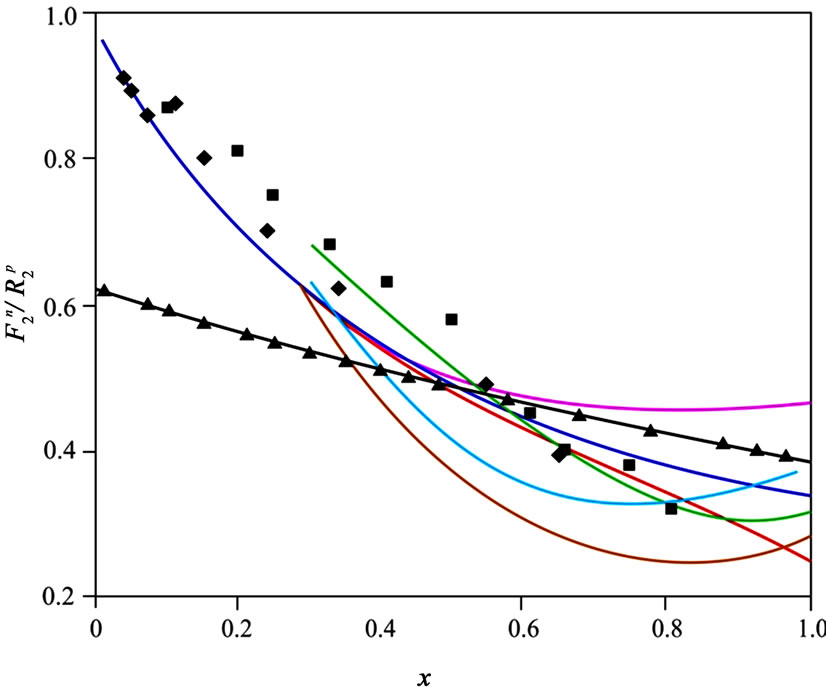
Plot taken from: A. Heidari and M. Ghorbani, "An Analytical and Numerical Approach to the Self-Consistent Method for Computing the Proportion of $F_2^n/F_2^p$ Using the 3He and 3H Nucleuses’ Structure Function and EMC Ratio," Journal of Modern Physics, Vol. 3 No. 1, 2012, pp. 124-128. doi: 10.4236/jmp.2012.31017. (Open Access link here)
