The flux is defined as integal {if you don't know calculus, take this as a sum} of $\int_\Gamma \vec B \dot \, \mathrm d \vec A$ where dA points out the surface $\Gamma$.
[![][1]][1]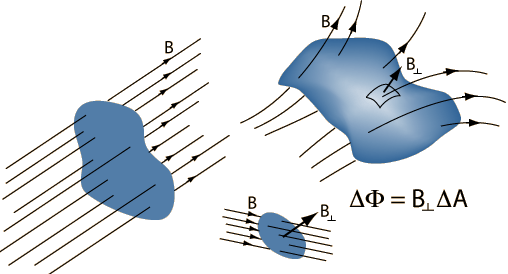
(source: gsu.edu)
if you had a circular shape of coil, the flux would not change, and hence, no Electric field or EMF would be induced, but here, as the field of solenoid may not be perfectly symmetrical outside it, (spreads out evenly in each direction) . hence, there is bound to be some emf, not too much, theoretically for an ideal solenoid, there would be no emf induced too. [1]: https://i.sstatic.net/M7WXF.gif
