It depends what you meanAs has been pointed out by @CuriousOne you can save.
This factor $\frac 1 2$ comes into play the energy but let's me first explain the context in lotswhich one usually first meet this loss of other situations eg the energy stored.
A capacitor $C$ in an inductorseries with a resistor $\frac 1 2 LI^2$$R$ and in a springvoltage source $\frac 1 2 k x^2$$V$ is charged.
In all these casesIf the "missing" energy turns up as heat due tofinal charge on the resistance in an electrical circuitcapacitor is $Q$ then the work done by the voltage source is $VQ$ and friction inas $Q=CV$ the mechanical example.
So not to wastework done by the energy requires a system which does not have resistance or frictionsource is $CV^2$.
In the electrical case assume thatThe energy stored in the resistance is very low and you attempt to charge a capacitor from a voltage sourceis $\int_0^QVdQ = \int_0^Q\frac Q C dQ = \frac 1 2 CV^2$.
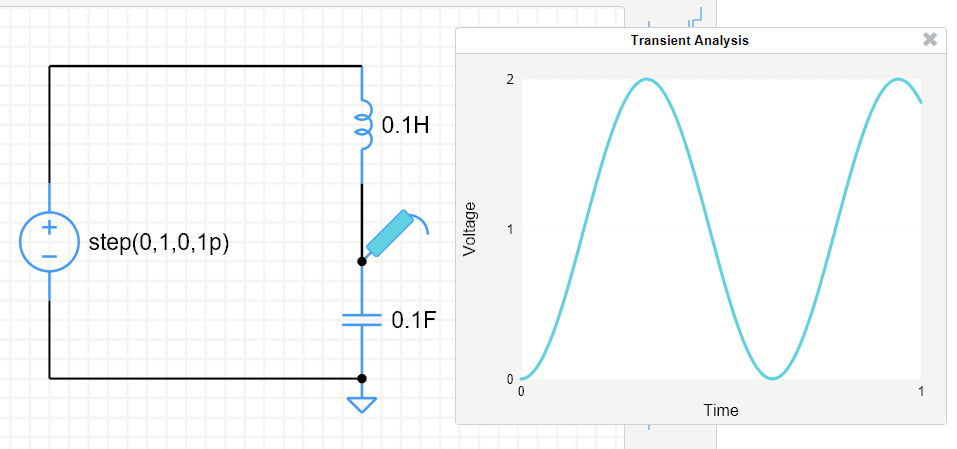
If The integration has to be done because as the resistancecapacitor is low enoughcharged the growth of voltage across the capacitor is not oscillatoryits plates changes.
The voltage across the capacitor becomes oscillatory about the supply voltagemissing energy is $\frac 12 CV^2$ and at times the voltage across the capacitor actually exceeds the voltage ofthat is lost as heat in the voltage supplyresistor.
ThisTo show that is because in such circumstancetrue and the inductanceloss is independent of the circuit (the loop which isvalue of the resistance in the circuit) becomes important and you have an LCR one must do a bit more circuit with voltages and currents oscillating at the circuits natural frequencyanalysis.
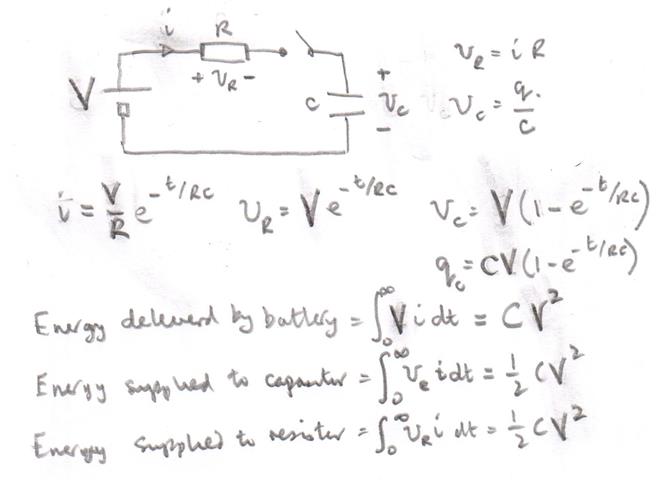
Because ofDuring the resistancecharging process it can be shown that the current in the circuit these oscillations will dampen down and the final state will be$I(t) = \frac V R e^{-\frac {t}{RC}}$.
The power dissipated in a resistor is $I^2R$ so during the capacitor storing halfchanging process the energy and the rest dissipated as heat.
But thatin the resistor is not all$\int_0 ^\infty \frac {V^2}{R^2} e^{-\frac {2t}{RC}} dt = \frac 1 2 CV^2$. An accelerating unbound
If the capacitor is charged particle emits electromagnetic radiationin the way described changing the resistance value will not change the amount of energy lost as heat.
SoIf the resistance in our example somebecomes very low instead of the "lost"charging process following an exponential curve the current in the circuit becomes a damped sinusoid and then energy will becomeis lost as heat and electromagnetic waves which are radiated out from the circuit. The smallerbecause the resistanceelectrons in the circuit the more this effectwires are accelerating and unbound accelerating charges emit electromagnetic radiation.
The factor $\frac 1 2$ comes into play whilstin lots of other situations eg the circuit is reaching steady stateenergy stored in an inductor $\frac 1 2 LI^2$ and in a spring $\frac 1 2 k x^2$.
And forIn all these cases the spring"missing" energy turns up as heat due to the resistance in an electrical circuit and friction in the mechanical example.
I imagineUsing Hooke’s law for a spring, of spring constant $k$ withwhich has a weight $mg$ on the end of it. If thestatic extension isof $x$ then $mg=kx$ - Hooke's Law.
However let's look at this in terms of energy.
Unextended spring andwhen a weight $mg$ is added to the thehung from its end and the spring extends by $x$ $mg= kx$.
Energy stored in spring is $\frac 1 2 kx^2$ and
The work done by gravitythe gravitational force is (loss of potential$mgx = kx^2$ whereas the energy) stored in the spring is $mg\cdot x = k x^2$$\frac 12 k x^2$
HalfIn this case imagine releasing the energymass when the spring is missingunextended.
Of course it is not. The rest of the energy isWhat would happen?
The mass would oscillate about the kineticstatic extension position and as frictional forces converted mechanical energy ofinto heat the mass as it passes throughwould eventually reach the static equilibrium position to eventually stop at an extension of $2x$. At this point work done by gravity = energy stored in springposition. $mg\cdot 2x = kx \cdot 2 x = \frac 12 k (2x)^2$
So catch hold of the spring at this point and
So how can you have allsave that "extra"missing energy available to you even though that factor of $\frac 12$ has turned up again.?
And of course, in principle, you can do the sameOne reason for introducing the capacitor.
Later in answer to a comment.
The equation ofspring is that perhaps discussing the motion forof a spring-mass system with resistance is of the same form as that formakes it easier to understand an LCR circuit. The inductor is the inertia (mass) component and the capacitor (or rather 1/C) has a resemblanceanswer to the spring constant - the springiness term.this question?
IfWhat you holding a mass atdo is, with the end of an unextended spring andunextended, release the mass what will happen? It all depends on the valuesand catch hold of the mass, the spring constant and the drag force when it first stops after half a period.
If the drag force is relatively small (the system is under damped) the mass
If there are no frictional forces this will overshoot its static equilibrium position and undergo damped harmonic and eventually come to rest at the static equilibrium position. Ifhappen when the extension at that point is $x$ then the energy stored inof the spring is $\frac 1 2 k x^2$ However if you move a mass down a distance $x$ the$2x$.
The work thatdone by the gravitational force does is now $mgx = kx^2$$mg 2x = 2kx^2$. The difference
The energy stored in the spring is $\frac 1 2 k x^2$$\frac 1 2 k (2x)^2 = 2 k x^2$ which is dissipated as heat dueexactly equal to the drag.
Ifwork done by the draggravitational force is very large (system over damped) then the mass again will reach the static equilibrium position but wiout oscillating again with a loss to the system of mechanical energy $\frac 1 2 kx^2$ which again becomes heat.
The charging capacitor is really an example of an LCR circuit where the small value of L makes results in this system being over damped and you get to the final state of the capacitor being charged without any oscillation. However again there is a loss ofNo energy to the system of $\frac 12 C V^2$ due to ohmic heatingwasted as heat.
Later still So what about the capacitor?
Let me start from the beginning.
There is no way thatHere you can chargeneed as small a capacitor without some resistance being presentvalue in the circuit as possible so a charging circuit for athat the voltage across the capacitor will always contain a battery, a resistoroscillated between 0 and a capacitor in series with one another as shown in2V about V (the final voltage value), just like the diagram belowspring.
When one analysis such a circuit one finds that the charging process does not occur instantaneously andIn this case you disconnect the relevant equations forcapacitor from the voltages, current and charge are listed below.
Energy is power $\times$ time and for electrical circuits power is voltage $\times$ time.
Because the voltages and currents are not constant to find the energy one has to integrate.
I have not shown details of the integration but have put downsource after half a period when the results ofvoltage across the integrationscapacitor is 2V.
You will note that the energyThe work done by the battery suppliesvoltage source is now $CV^2$$V 2Q = 2CV^2$.
The
The energy supplied tostored in the capacitor is $ \frac 1 2 CV^2$.
The energy supplied to the resistor$\frac 1 2 C (2V)^2 = 2 CV^2$ which ends up as heat is $ \frac 1 2 CV^2$. This is where the "missing" energy has gone.
An important thing aboutexactly equal to the last statement is thatwork done by the voltage source.
No energy lostwasted as heat does not depend on the resistance of the resistance.
 You have all the energy supplied by the voltage source stored in the capacitor.
You have all the energy supplied by the voltage source stored in the capacitor.