The baryon non-conservation in the quantum theory is caused by the chiral anomaly, which leads to the baryon current $j_B^\mu = \sum_i \bar q_i \gamma^\mu q_i$ being not conserved as $$ \partial_\mu j_B^\mu \propto F\wedge F$$ where $F$ is the field strength tensor of the electroweak field. Now, $\int \partial_\mu j_B^\mu \propto \int F\wedge F$ is a topological invariant, associated to the instanton (see also) sector we're in. The instanton is essentially the classical solution - the vacuum - we're basing our quantum field theoretic perturbative expansion around (see also thisthis and thisthis post of mine). This means instantons are invisible from the perturbative viewpoint - you have to fix one to do perturbation theory.
Furthermore, if one expands the partition function as a Taylor series around vanishing coupling constant, which essentially defines the perturbative approach, the instanton contributions vanish because they are suppressed with a factor $\mathrm{e}^{-1/g}$ whose Taylor expansion at vanishing coupling is identically zero, and hence doesn't appear in the perturbation series (cf. nLab).
Graphically, the anomaly and hence the non-conservation is associated to the triangle diagrams
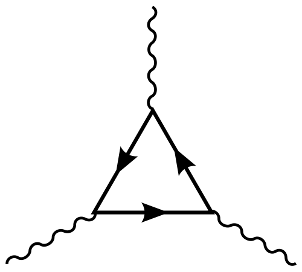
see "Lectures on Anomalies" by Bilal.
