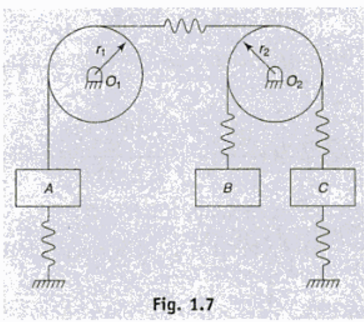
In the above mechanism it is required to find the generalized coordinates to write equation of motion of the MDOF system. I just want to ask how do we actually approach such a problem? How to identify which kinematic degrees of freedom are relevant and independent?
The answer given in this particular case is 4$4$. Two rotation of the pulleys, and two vertical motions of the masses B$B$ and C$C$. Now how to visualize this? How do I methodically come to this conclusion? Again, is there any other combination possible?
All pulleys are masslessmass-less and frictionlessfriction-less.