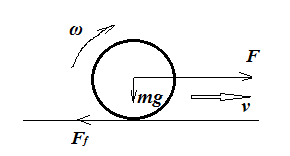
Consider the diagram below of a ball on a horizontal surface:
Newton's Laws tell us that if no net force acts on the ball it will remain in a constant state of motion ($v=0$ or $v=\text{constant}$). Consequently, if no net torque acts on the ball its state of rotation will also remain constant ($\omega=0$ or $\omega=\text{constant}$).
Where friction does play a part, it is usually somewhat simplistically modelled as $F_f=\mu F_n$ with $\mu$ some friction coefficient and $F_n$ the normal force (here simply $mg$). But in the case of a ball rolling with constant $v$ and constant $\omega$ and no external force (say $F$) acting on it, this would lead to deceleration according to :
$$ma=-F_f$$
But then $F_f$ would also provide torque leading to angular acceleration according to:
$$I\dot{\omega}=F_f R,$$
with $I$ the inertial moment, $R$ the radius and $\dot{\omega}=\frac{d\omega}{dt}$ the angular acceleration.
This would be the case where you launch a ball with initial speed $v$ but no angular momentum ($\omega=0$) onto a surface that provides much friction: the ball would start spinning ($\dot{\omega} > 0$ but also start decelerating ($a < 0$) and translational kinetic energy would be converted to rotational kinetic energy. If the surface can provide enough friction that process would continue until $v=\omega R$: rolling without slipping.
To keep the ball moving without any deceleration we would have to supply an external force, so that:
$$F_f=F,$$
and $a=0$.
Critical coefficient of friction $\mu_c$:
Assume a ball with $v=0, \omega=0$ at $t=0$. We now apply a horizontal force $F$, so that $a>0$.
$$F_f=\mu F_n=\mu mg$$
$$I\dot{\omega}=F_fR=\mu mgR$$
After integration we get:
$$\omega=\mu\frac{mgR}{I}t$$
In that same amount of time the ball has also acquired translational speed:
$$F-F_f=ma$$
After integration we get:
$$v=\frac{F-\mu mg}{m}t$$
Without slipping we have:
$$v=\omega R$$
$$\frac{F-\mu mg}{m}t=\mu\frac{mgR^2}{I}t$$
Reworking and isolating $\mu$ we get:
$$\large{\mu_c=\frac{FI}{mg(I+mR^2)}}$$$${\mu_c=\frac{FI}{mg(I+mR^2)}}$$
This is the minimum value for the coefficient of friction in order to achieve rolling without slipping when a horizontal force $F$ is applied.