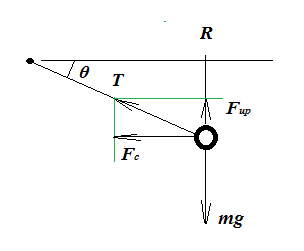
We have the ball orbiting at a distance $R$ from the centre of rotation and the string inclined at angle $\theta$ with respect to the horizontal.
Two main forces act on the ball: gravity $mg$ ($m$ is the mass of the ball, $g$ the Earth's gravitational acceleration) and $F_c$, the centripetal force needed to keep the ball spinning at constant rate. $F_c$ is given by:
$$F_c=\frac{mv^2}{R},$$
where $v$ is the orbital velocity, i.e. the speed of the ball on its circular trajectory.
Trigonometry also tells us that if $T$ is the tension in the string, then:
$$T\cos\theta=F_c.$$
Similarly, withas the ball is not moving in the vertical direction, thus $F_{up}$:
$$T\sin\theta=F_{up}=mg.$$
From this relation we can infer:
$$T=\frac{mg}{\sin\theta}.$$
And so:
$$\frac{mg}{\tan\theta}=F_c=\frac{mv^2}{R}.$$
Or:
$$\tan\theta=\frac{gR}{v^2}.$$
From this follows that for small $\tan\theta$ and thus small $\theta$ we need large $v$. But at lower $v$, $\theta$ increases. Also note that $\theta$ is invariant to mass $m$.