If by "they are associated with different masses" you mean that the flavor eigenstates have different masses then you are working from a misconception. Those states are not eigenstates of the free Hamiltonian so they don't have a mass as such. (They do have a mean expectation if you could weight a bunch of them, but it does not apply to any given neutrino.)
I tried to address the situation somewhat in the last paragraph of my answer to the question that Qmechanic linked in the comments.
The neutrinos are generated byUpdate 30 April 2012 I had a weak interaction intalk with Fermilab theorist Boris Kayser today after he gave a pure flavor state. That said they instantly start propogating which means we project into the mass basis where we havecolloquium and he squared me away on a sum of three eigenfunctions $$ |initial\rangle = \sum_i U_{\beta,j} |\mu\rangle$$ which all exist in the same part of spacefew things.
This question is one that has been considered many times by many people in many ways.
Not only is what I had written originally not rigorous, but attempts to make it rigorous run into real trouble and get a result at odds with the conventional formalism and inconsistent with experiment.
There is a way to make a rigorous analysis (whole thing at arXiv:1110.3047), and it ends up agreeing with the usual formulation at first order in $\Delta m^2_{i,j}$. It requires that you consider an experiment in the rest frame of the particle that decays to produce the neutrino (and a charged lepton). You define that decay as occurring at space time point $(0,0)$ and compute the amplitude for a neutrino in mass state $i$ to be detected at space time point $(x_\nu, t_\nu)$ in coincidence with the charged lepton being detected at space time point $(x_l, t_l)$ (both also written in the rest frame of the decay particle). Then you notice that the propagators for the two leptons are kinematically entangled. Sum the amplitudes coherently (because the mass state of the neutrino is unobserved). Using the fact that the neutrinos are ultra-relativistic approximate to first order in $\delta m^2_{i,j}$ and drop all terms that don't affect the phase-differences (because neutrino mixing only depends on the phase differences). Somewhere in there was a boost back to the lab frame and a cute calculation of how L-over-E is invariant under the boost: $\frac{L^0}{E^0} = \frac{L}{E}$. The result should be the one we usually give, only now we've dealt with this cute little puzzle.
Now in principle each of these has a well defined energy and momentum and will start propagating at a speed consistent with them, soHere's an image from an earlier talk he gave on the wave function insame subject which shows the three mass states initially overlapped will begin to spread out.
And already we're in territory forwhole process on which the quantum philosophers because we can askcalculation is performed:
"Shouldn't the energy and momentum from the interaction depend on the mass, and if the neutrino goes long distances how will that compare to the time of flight (given that in time the mass parts of the wave packet could become completely uncoupled)?"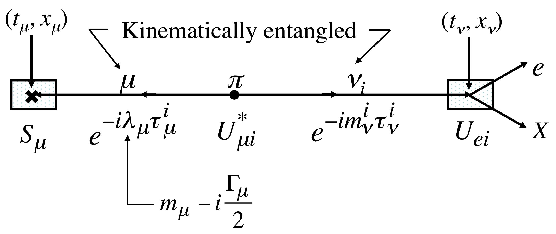
My answerSo, long story short: Good question, the usual formalism doesn't seem to those questions arehave a good answer, but a rigorous calculation "I don't know"can. It is the kind of thing that makes either consistent histories interpretations or some kind of decoherence mechanism (to make these decisions on a more local basis) very appealing.
An important note in all this is that all the neutrinos we observe are ultra-relativistic (with energies millions of times their masses), so it would be very difficult for us to experimentally verify this picturemade and at this timeto leading order agrees with the usual formalism.
