Indeed, the emf in one half of the solenoid will cancel that in the other half. This is most easily visualized if you split the solenoid in half, now you have one solenoid that is being entered, and one that is being exited so their EMF will be equal and opposite.
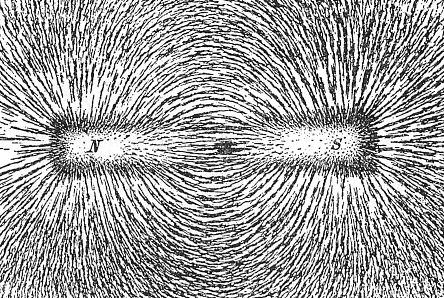
Or if you're looking at the center only, all of the flux lines in the middle are parallel to the motion, so there will be no change in flux.
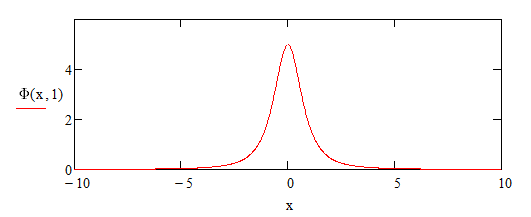
So if you look at a plot of number a field lines encircled by a ring at a given position (which is proportional to the flux) you'll get something that looks like:
As you can see there is a maximum amount of flux right in the middle, and on either side it is symmetrically decreasing and increasing. The EMF produced by a coil is proportional to the change in flux with time. If the magnet/coil is moving at a constant velocity, that means the EMF will be proportional to the change in flux with position.
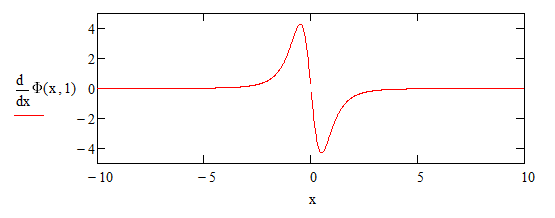
Here is the derivative of the flux with respect to position. This would be proportional to the EMF that an infinitely thin coil would experience as a magnet passed through it.
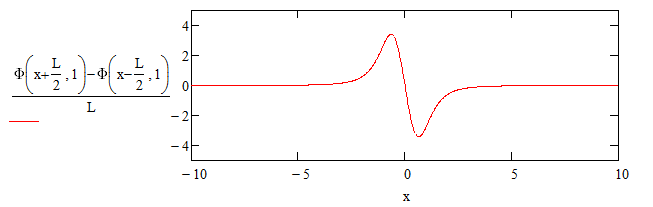
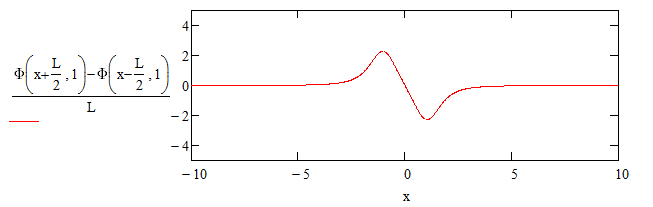
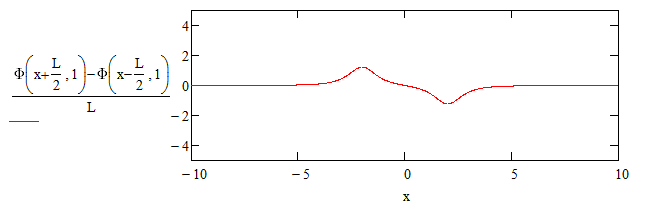
If the coil had some width to it, we would want the average change in flux over the whole coil. To take the average one can integrate and divide by the length, but since we'd be integrating the derivative of the flux, we can just take the difference between the endpoints.
For relatively thin coils this ends up looking almost identical to the infinitely thin coil, but as the coil length increases the graph does begin to look different. However, the middle must always pass through zero because the graph of the flux is symmetric (because the magnetic field is symmetric, so however much the EMF is positive on one side it will exactly cancel the negative on the other side).
$$ $$
All charged particles feel a force when passing through a magnetic field. When the wire doesn't form a loop it's necessary to calculate the EMF via the Maxwell–Faraday equation instead of Lenz's Law.