\begin{equation} \mathbf{a}=\dfrac{\Delta \mathbf{v}}{\Delta t} \tag{01} \end{equation}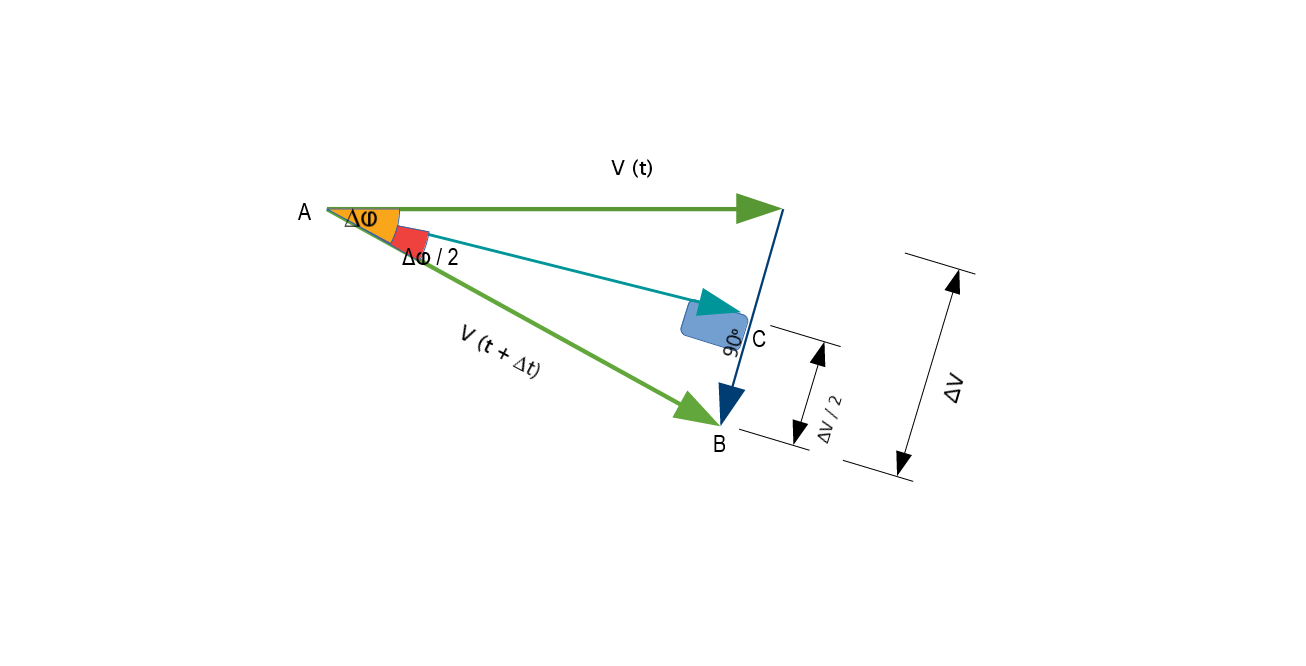 \begin{equation} \vert\mathbf{a}\vert=\dfrac{\vert \Delta \mathbf{v} \vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta \phi\vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta s\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon \cdot \upsilon \vert\Delta t\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon^{2}}{r} \tag{02} \end{equation}

The Centripetal Acceleration vector $\mathbf{a}$ is pointing inwards to the center, as shown in Figure (vector $\Delta \mathbf{v}$)

In right-angled-triangle ABC \begin{equation} \dfrac{|\dfrac{\Delta \mathbf{\vec v}}{2}|}{|\vec V|}=Sin\dfrac{\Delta \vec \theta}{2} \tag{01} \end{equation} If \begin{equation} \theta \tag{02} \end{equation} is small \begin{equation} v(t) \approx v(t + \delta t ) = \mathbf{\vec v} \tag{03} \end{equation} \begin{equation} \dfrac{|\dfrac{\delta \mathbf{\vec v}}{2}|}{|\vec V|}=Sin\dfrac{\delta \vec \theta}{2} \tag{03} \end{equation} For small angle \begin{equation} \delta \theta \approx Sin {\delta \theta} \tag{04} \end{equation} So on rearranging \begin{equation} \dfrac{\delta \mathbf{\vec v}}{2} =\dfrac{\delta \vec \theta}{2} \times \vec v \tag{05} \end{equation} \begin{equation} \delta \mathbf{\vec v} =\delta \vec \theta \times \vec v \tag{06} \end{equation} \begin{equation} \dfrac{\delta \mathbf{\vec v}}{\delta \mathbf{t}} =\dfrac{\delta \vec \theta}{\delta \mathbf{t}} \times \vec v \tag{07} \end{equation}

\begin{equation} \mathbf{\vec a}=\dfrac{\delta \mathbf{\vec v}}{\delta \mathbf{t}} \tag{08} \end{equation}

\begin{equation} \vec \omega =\dfrac{\delta \vec \theta}{\delta \mathbf{t}} \tag{09} \end{equation}

\begin{equation} \mathbf{\vec a}=\vec \omega \times \vec v \tag{10} \end{equation}

\begin{equation} \mathbf{a}=\omega \times v \tag{11} \end{equation} And since \begin{equation} \mathbf{v}=\omega \times r \tag{12} \end{equation} So \begin{equation} \mathbf{a}=\dfrac {v^{2}}{\mathbf{r}} \tag{13} \end{equation}

\begin{equation} \mathbf{a}=\dfrac{\Delta \mathbf{v}}{\Delta t} \tag{01} \end{equation} \begin{equation} \vert\mathbf{a}\vert=\dfrac{\vert \Delta \mathbf{v} \vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta \phi\vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta s\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon \cdot \upsilon \vert\Delta t\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon^{2}}{r} \tag{02} \end{equation}

\begin{equation} \mathbf{a}=\dfrac{\Delta \mathbf{v}}{\Delta t} \tag{01} \end{equation} \begin{equation} \vert\mathbf{a}\vert=\dfrac{\vert \Delta \mathbf{v} \vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta \phi\vert}{\vert\Delta t\vert}=\dfrac{\upsilon \vert\Delta s\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon \cdot \upsilon \vert\Delta t\vert}{r \vert\Delta t\vert}=\dfrac{\upsilon^{2}}{r} \tag{02} \end{equation}

The Centripetal Acceleration vector $\mathbf{a}$ is pointing inwards to the center, as shown in Figure (vector $\Delta \mathbf{v}$)