Imagine a box where each side has an area $A$, and suppose that it contains a continuous flow of rain that falls vertically at a velocity $u$. The density of raindrops is $\rho$ (that's the number of drops per unit volume).
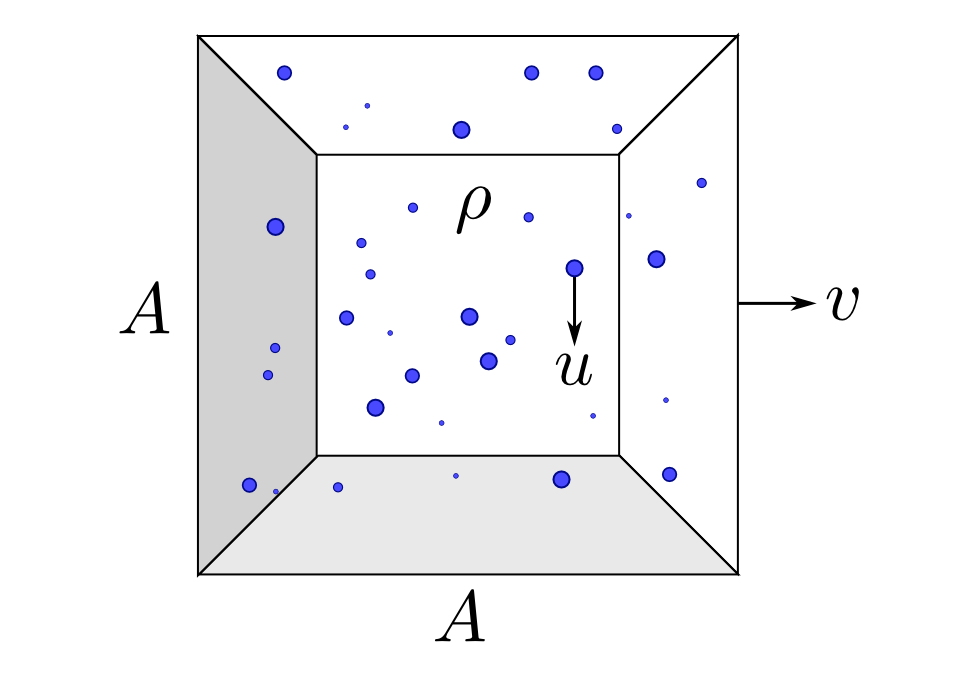
The rate at which the drops hit the bottom panel will be $\rho Au$ and the rate they hit the vertical (left) panel will be $\rho Av$, giving a total rate
$$ R=\rho A(u+v) $$
In the case of a car, you have a single windshield of total area $A$ at an angle $\theta$. Its horizontal cross-section is $A\cos\theta$ and its vertical cross-section is $A\sin\theta$. So the total rate will be
$$ R=\rho A(u\cos\theta + v\sin\theta) $$
where $v$ is the speed of your car.
Edit. The above comments that claim the same volume of rain hits your car for a given distance, irrespective of speed, is wrongare misleading. The longer you travel for, the more rain falls on your windshield. But the volume that you pick-up from moving is indeed only dependent on total distance travelled:
\begin{align} N&=\int_0^T R(t)\,dt \\ &=\rho A \left(uT\cos\theta + \sin\theta \int_0^T v(t)\,dt\right) \\ &=\rho A(uT\cos\theta + d\sin\theta) \end{align}
