As a side note skidding is not a yes or no state with tires. See this answerthis answer for more details.
The sum of the normal force and friction force that act on the car is the reaction force to the sum of the weight and centrifugal force of the car on the road.
We can equate them in the coordinate frame parallel and perpendicular to the road.
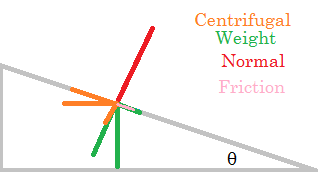
$$N=m\,g\, \cos(\theta) + m\,r\,\omega^2\,\sin(\theta)$$
In order for the car to not skid the maximum available frictional force must exceed the virtual centripetal force in the direction parallel to the road.
$$\mu_s\, N \gt |m\,r\,\omega^2\,\cos(\theta)-m\,g\, \sin(\theta)|$$
Combining yields:
$$\mu_s (m\,g\, \cos(\theta) + m\,r\,\omega^2\,\sin(\theta)) \gt |m\,r\,\omega^2\,\cos(\theta)-m\,g\, \sin(\theta)|$$
$$\mu_s \gt \frac{ |r\,\omega^2\,\cos(\theta)-g\, \sin(\theta)|}{g\, \cos(\theta) + r\,\omega^2\,\sin(\theta)}$$
