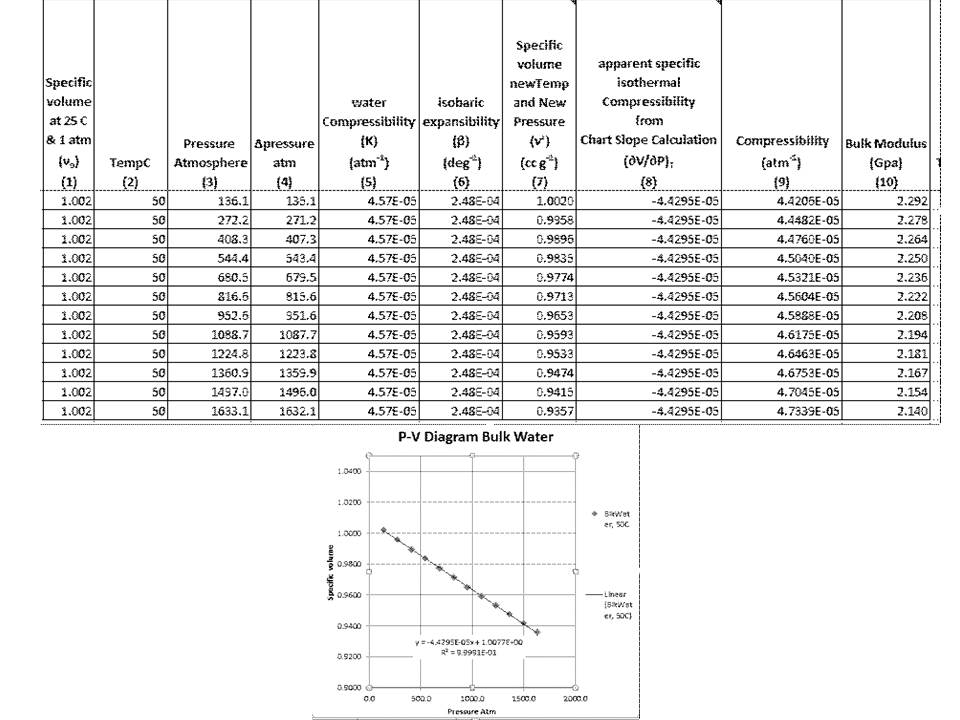
I simply want to calculate the bulk modulus of water at 50C and increasing pressures. I think I am correctly calculating the new specific volume from the original conditions at (25C and 1atm) to 50C and higher pressures. I am rightly getting a decrease in specific volume with increasing pressure at constant temperature (Column7). Here is the spreadsheet:
${V}^{'}={V}_{o}e^{{\beta}(T-25)-\kappa\Delta P}$
where:
${V}^{'}$ is column 7
${V}_{o}$ is column 1, the specific volume of water at 1 atm and 25C
$T$ is in Celsius
$P$ is in atm.
I used the above cross plot to graphically solve the slope $(\frac{\partial v} {\partial P}) $$(\frac{\partial v} {\partial P})_{T} $ and input it into Column 8:
Then to calculate the new compressibility at 50C (${\kappa}$) Column 9:
${\kappa}=-\frac{1} {V}\frac{\partial v} {\partial P} $${\kappa}=-\frac{1} {V}(\frac{\partial v} {\partial P})_{T} $
which gives me the new compressibiliy Column 9. Then I I just take the reciprocal and convert the units to GPa.
Oops, bulk modulus (Column 10) should be increasing with pressure at a constant temperature, not decreasing. I know that since dividing by an ever decreasing specific volume as pressure increases will give me a larger compressibility (Column 9) and a decreasing Bulk Modulus (Column 10). But everyone knows increasing pressure should have the opposite effect. Where did I go wrong?
