Here's a solution which is similar to John Rennie'sJohn Rennie's but hopefully less involved. I'll steal his image, too:
The pendulum has kinetic energy $T$, potential energy $U$, and total energy $E=T+U$, where $$ T = \frac12 m\ell^2 \dot\theta^2, \quad\quad U = mg\ell(1-\cos\theta). $$
The simple harmonic approximation takes the limit $\theta\ll1$, where $$ U = mg\ell \left( \frac{\theta^2}{2!} - \frac{\theta^2}{4!} + \cdots \right) \approx mg\ell \frac{\theta^2}2 \equiv U_\text{quadratic} $$ Now it's clear at small $\theta$, and happens to be the case for all $\theta$, that $U_\text{quadratic}$ is an overestimate of $U$:
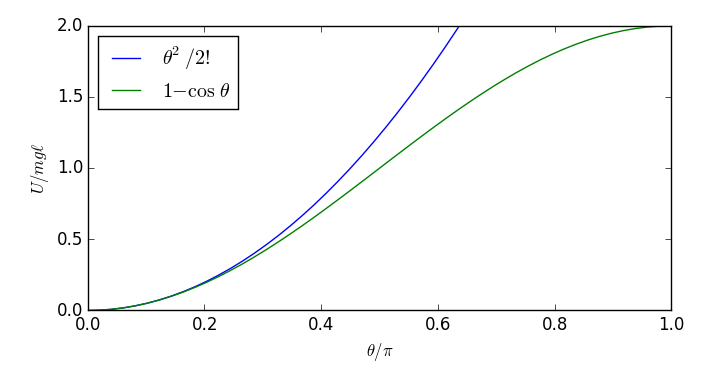
Therefore whatever our starting $\theta$ happens to be, using the simple harmonic approximation $U_\text{quadratic}$ predicts too much total energy $E$, and correspondingly too much kinetic energy $T$ --- our physical pendulum goes slower than in the approximation. The prediction of a constant period $\tau_\text{quadratic} = 2\pi\sqrt{\ell/g}$ is therefore an underestimate, and the underestimate gets worse for large amplitudes, so the period must increase with amplitude.
