LIGO has now observed gravitational wavesLIGO has now observed gravitational waves, so the theory for it working is clearly sound.
The light path itself is also affected by the gravitational wave. The Wikipedia article on LIGOLIGO says,
Note that the effective length change and the resulting phase change are a subtle tidal effect that must be carefully computed because the light waves are affected by the gravitational wave just as much as the beams themselves.
Wikipedia then provides a link to Chapter 27 of Kip Thorne's lecture series on Classical Mechanics. Section 27.6 of that Chapter covers, in great detail, how LIGO would actually work. In the idealized model, the gravitational waves (as seen in a local Lorentz frame of LIGO) change the length of the arms by an amount $\delta x=\frac12h_+\ell_x$ and $\delta_y=-\frac12h_+\ell_y$ where $h_+(t)$ is the gravitational wave (i.e., the increase in $x$ length is coincided by a decrease in the $y$ length of the same amount). The phase caused by this difference can be calculated to be $$ \Delta\varphi(t)=\omega_0\,2\left(\delta x-\delta y\right)=\omega_0\left(\ell_x+\ell_y\right)h_+(t) $$ where $\omega_0$ is the light's angular frequency (and $c=1$).
Since there is a phase shift, then the light intensity being sent to the photodetectors are also being modified, with the intensity linearly proportional to the phase shift, which leads it to being directly proportional to the gravitational wave: $$ \Delta I_{PD}(t)\propto\Delta\varphi(t)=2\omega_0\ell h_+ $$ where we've assumed $\ell_x\approx\ell_y\equiv\ell$. There is a bit more detail involved in getting a real signal from a real set up (e.g., accounting for local gravity), but the basis for LIGO is essentially the above.
Note that the phase shift itself isn't caused by the lengthening and shortening of the paths because the wavelength of light also changes by this factor. Instead, it is measuring the arrival time of the crests and troughs of the light wave between the two arms (cf. this LIGO Science Collaboration FAQ pagethis LIGO Science Collaboration FAQ page or this Physics World article on the discoverythis Physics World article on the discovery), so it is more of a stopwatch than a ruler.
Thorne's notes also show the predicted signal LIGO should make,
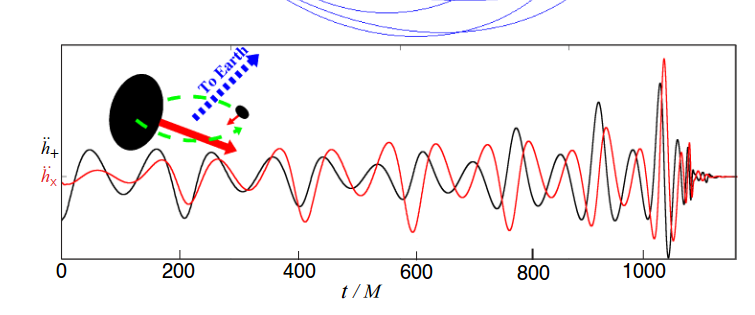
which looks remarkably like the real signal
real signal http://static4.techinsider.io/image/56bcad01dfb6b41877f420f8-950-490/screen%20shot%202016-02-11%20at%2010.45.59%20am.png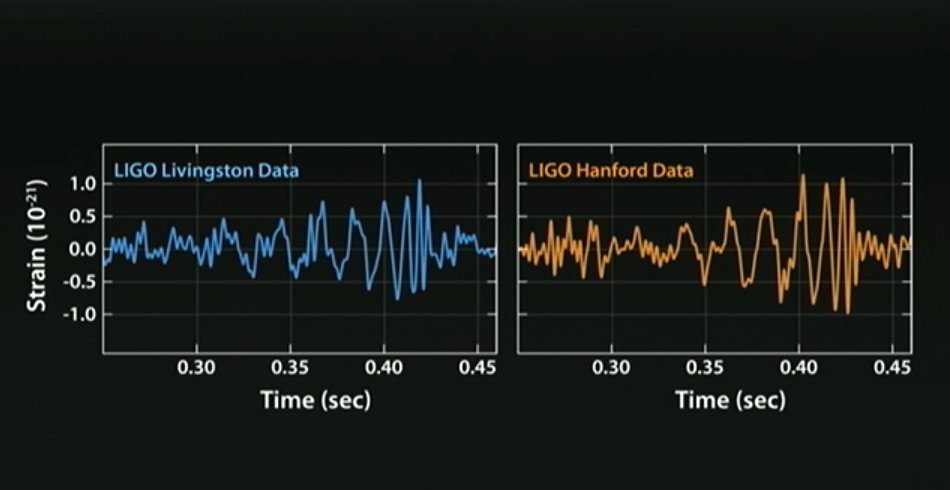
(sourcesource)
