The relevant equation is that of complex power in an AC circuit:
$$\vec{S}=\frac{\vec{V} \vec{I}^{*}}{2}=\frac{V I}{2} \angle -\phi$$
This is to say, the complex power flowing through a line is equal to the complex voltage times the conjugate of the complex current divided by two. Those not familiar with AC circuits will have a tough time with the complex variables, but let's turn to the following definitions for illustration. Note that I'm going to establish voltage as the reference angle.
$$S=P+jQ$$ $$\vec{I}=I \angle \phi$$ $$\vec{V}=V \angle 0^{\circ}$$
The real power is $P$ and the imaginary power is $Q$. The interesting thing to note is that $P$ can be either negative or positive. Before applying any of this however, you must have a reference direction. You're looking at the line, you can look either right or left, you establish one of those directions as positive, and then $P$ will follow as either negative or positive and if positive, it flows in the direction you're looking.
Here is an illustration, although I think this differs in convention by the sign of $\phi$.
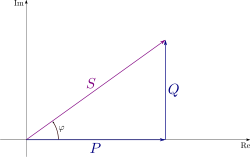
Now, here is what you need to establish the direction of power flow:
- A reference direction
- The angle between the voltage and current
Exactly how you measure this is certainly the difficult part. I will defer to other answer regarding that. But provided that you do, if $\phi \in (0,\pi)$$\phi \in (-\pi/2,\pi/2)$ then the power flows in the positive direction and if $\phi \in (-\pi, 0)$$\phi \in (\pi/2, 3\pi/2)$ it flows in the negative direction.
