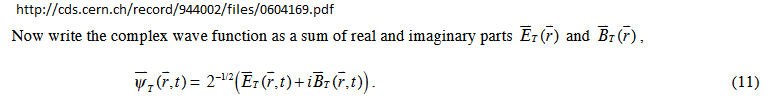Edit after this comment:

can you please tell me if the photon has no EM charge, why does it still interact with the electric field of a charge (like and electron), via virtual photons? Does it attract or repel? I understand that when a photon interacts with the electric field of a charge (like an electron), it is not the same as when an electron interacts with a proton, because they attract. Neither like when two electrons interact, they repel. What does the electron's electric field do to the photon, does it repel or attract it? – Árpád Szendrei

The mistake is thinking in terms of electric field at the quantum level. The classical electric and magnetic fields are emergent quantities from the underlying quantum mechanical quantized Maxwell equations. "Emergent" means there is not one to one correspondance of the variables at the quantum mechanical level with the variables the one measures at the classical macroscopic level. Only demonstrable mathematical continuity.

Take the example of thermodynamics where the variables emerge from the underlying statistical mechanics variables, example: the temperature is related to the mean kinetic energy.

The classical fields can be seen to emerge mathematically from the quantum mechanical fields here.

In particular the photon obeys a quantized Maxwell equation and the E an B fields are connected to the mean values of the classical light

BUT within the wave function, which is a complex one and can only have a meaning as a probability distribution after interactions.

the questions in the comments:

Can you please tell me if the photon has no EM charge, why does it still interact with the electric field of a charge (like and electron), via virtual photons?

From the above you must understand that the classical electric field does not play a role at the quantum level, it will emerge at the classical level after integration over the quantum mechanical variables, similar as to how temperature can be defined as emergent from the underlying statistical mechanics level. It is because the same differential equation is being used for classical light and the description of a photon that the variables can be identified with the same symbol, E and B and convoluted for large dimensions to the classical fields. The interactions of the photon are perfectly described by quantum field theory, giving compton etc scatterings.

Does it attract or repel?

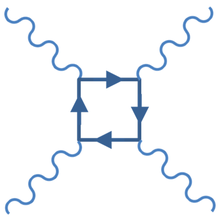
Depending on the sums of the Feynman diagrams it either scatters off , ( repel) or contributes to the attraction between charges as a virtual photon.

I understand that when a photon interacts with the electric field of a charge (like an electron), it is not the same as when an electron interacts with a proton, because they attract. Neither like when two electrons interact, they repel.

The photon, a quantum mechanical entity with a wavefunction describing it,interacts with an electron, another quantum mechanical entity described by a wavefunction. The electric field has no meaning for the electron. The photon will interact with the potential, which appears in the quantum mechanical framework, as in the hydrogen atom: one uses the 1/r potential, not the electric field.

The photon just has energy and spin, and only virtually a value for electric and magnetic field, in its wave function.

What does the electron's electric field do to the photon, does it repel or attract it?

It does nothing directly, only mathematically through the integrals involved in the scatter where the values become real and measurable as an interaction, as described in the original answer. Repulsion or attraction will depend on the initial values and other boundary conditions in calculating the crossections.

This link says why photon electric field interactions are "trivial" :

In a vacuum, the classical Maxwell's equations are perfectly linear differential equations. This implies – by the superposition principle – that the sum of any two solutions to Maxwell's equations is yet another solution to Maxwell's equations. For example, two beams of light pointed toward each other should simply add together their electric fields and pass right through each other. Thus Maxwell's equations predict the impossibility of any but trivial elastic photon–photon scattering. In QED, however, non-elastic photon–photon scattering becomes possible when the combined energy is large enough to create virtual electron–positron pairs spontaneously, illustrated by the Feynman diagram in the figure on the right.

A Feynman diagram (box diagram) for photon–photon scattering; one photon scatters from the transient vacuum charge fluctuations of the other

There exists a lower value of the electric field , the Schwinger limit, over which these interactions can occur and a photon in a strong electric field will interact with it with virtual lines and become scattered with a change in energy, Compton scattering.

For low electric fields there could be just an elastic scattering, i.e. Thomson scattering where it changes direction due to virtual exchanges and thus light built up by these photons can get an induced polarization.

At the photon level these four photon interactions have four electromagnetic , 1/137, vertices which means that with respect to first order interactions they are very much diminished. One needs the emergent beam from a huge number of photons to see the effect.

So the interaction with the electric field is very much different than with the gravitational field , where, as the other answer states, it follows General Relativity rules. The black hole charged or not distorts the curvature and defines the geodesics that the photons follow, as with gravitational lensing. There do exist studies of how, if the black hole is charged, photons could be further affected but that is a different story and not well defined yet.

This link says why photon electric field interactions are "trivial" :

In a vacuum, the classical Maxwell's equations are perfectly linear differential equations. This implies – by the superposition principle – that the sum of any two solutions to Maxwell's equations is yet another solution to Maxwell's equations. For example, two beams of light pointed toward each other should simply add together their electric fields and pass right through each other. Thus Maxwell's equations predict the impossibility of any but trivial elastic photon–photon scattering. In QED, however, non-elastic photon–photon scattering becomes possible when the combined energy is large enough to create virtual electron–positron pairs spontaneously, illustrated by the Feynman diagram in the figure on the right.

A Feynman diagram (box diagram) for photon–photon scattering; one photon scatters from the transient vacuum charge fluctuations of the other

There exists a lower value of the electric field , the Schwinger limit, over which these interactions can occur and a photon in a strong electric field will interact with it with virtual lines and become scattered with a change in energy, Compton scattering.

For low electric fields there could be just an elastic scattering, i.e. Thomson scattering where it changes direction due to virtual exchanges and thus light built up by these photons can get an induced polarization.

At the photon level these four photon interactions have four electromagnetic , 1/137, vertices which means that with respect to first order interactions they are very much diminished. One needs the emergent beam from a huge number of photons to see the effect.

So the interaction with the electric field is very much different than with the gravitational field , where, as the other answer states, it follows General Relativity rules. The black hole charged or not distorts the curvature and defines the geodesics that the photons follow, as with gravitational lensing. There do exist studies of how, if the black hole is charged, photons could be further affected but that is a different story and not well defined yet.