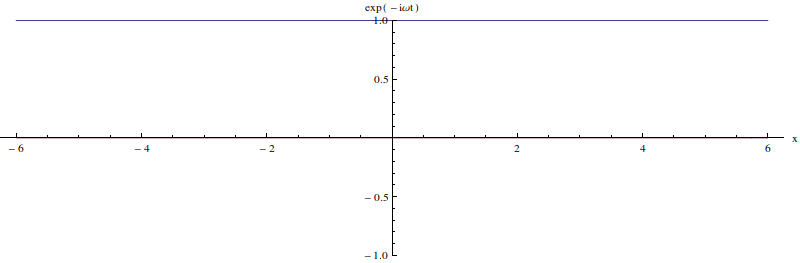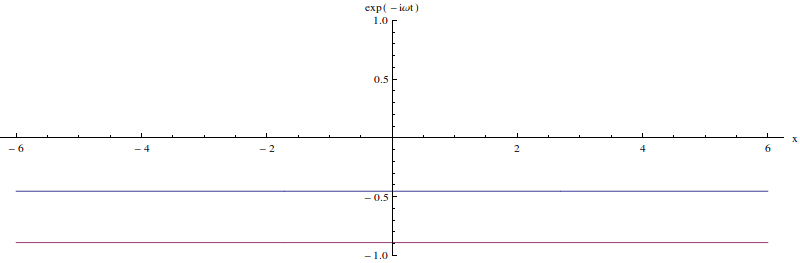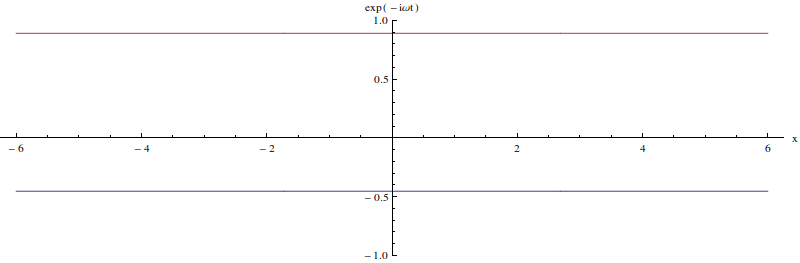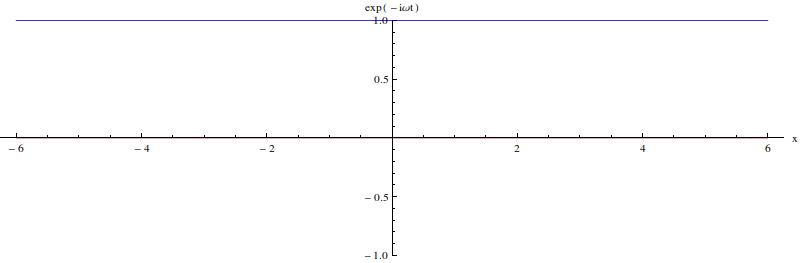A picture is worth a thousand words. Here's how it looks as a function of space, evolving in time:
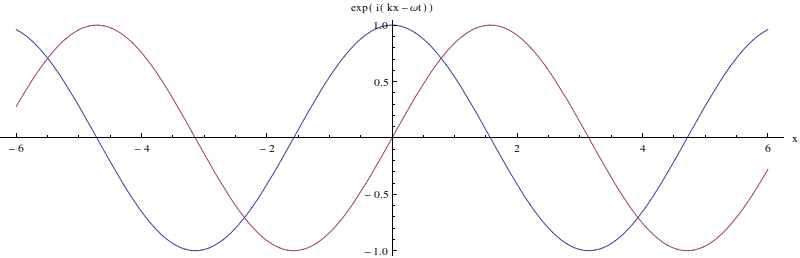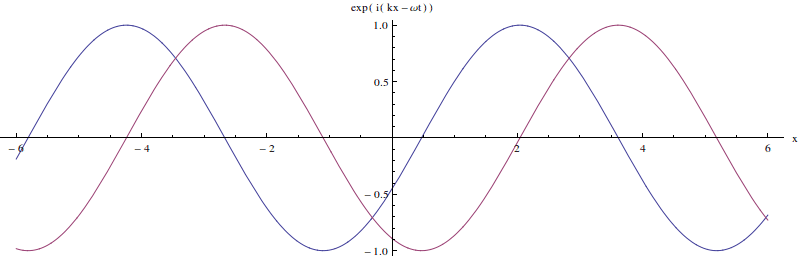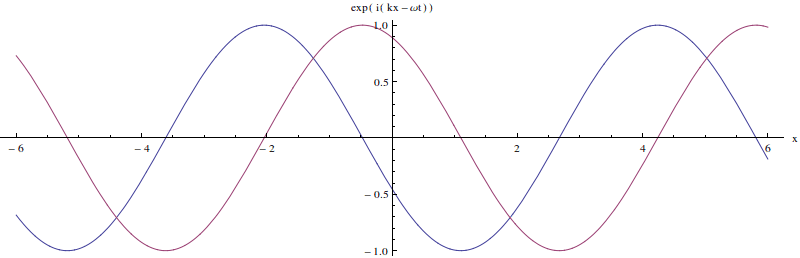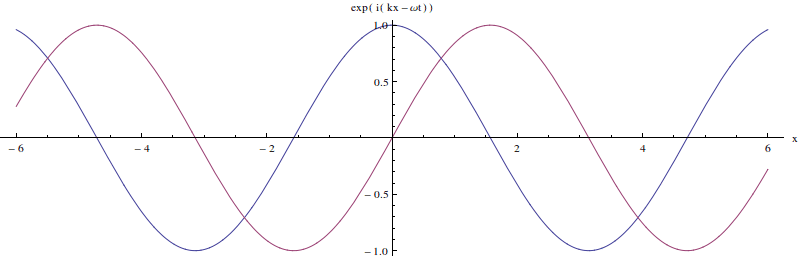
Here blue is real part, and purple is imaginary part of the complex exponent $\exp(i(kx-\omega t))$.
If you instead just look at $\exp(-i\omega t)$, you'll get this: