How is the momentum operator derived in Dirac formalism? I am reading Quantum Mechanics by Sakurai and he gives the following derivation. But I don't understand how he goes from the third equation to the last equation in (1.7.15). What I don't understand is where the partial derivative with respect to $x^{\prime}$ comes from. Here is the derivation from the book. 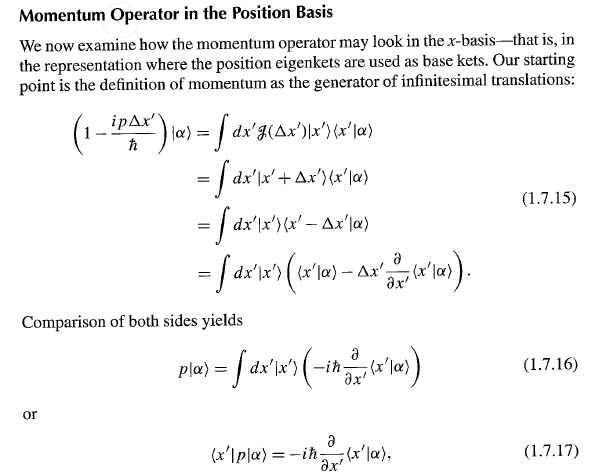
Momentum Operator in the Position Basis
We now examine how the momentum operator may look in the $x$-basis - that is, in the representation where the position eigenkets are used as base kets. Our starting point is the definition of momentum as the generator of infinitesimal translations:
$$\begin{align} \biggl(1 - \frac{ip\Delta x'}{\hbar}\biggr)\lvert\alpha\rangle &= \int dx' \mathcal{J}(\Delta x')\lvert x'\rangle\langle x'\lvert\alpha\rangle \\ &= \int dx' \lvert x' + \Delta x'\rangle\langle x'\lvert\alpha\rangle \\ &= \int dx' \lvert x'\rangle\langle x' - \Delta x'\lvert\alpha\rangle \\ &= \int dx' \lvert x'\rangle\biggl(\langle x'\lvert\alpha\rangle - \Delta x'\frac{\partial}{\partial x'}\langle x'\lvert\alpha\rangle\biggr).\tag{1.7.15} \end{align}$$
Comparison of both sides yields
$$p\lvert\alpha\rangle = \int dx'\lvert x'\rangle\biggl(-i\hbar\frac{\partial}{\partial x'}\langle x'\lvert\alpha\rangle\biggr)\tag{1.7.16}$$
or
$$\langle x'\rvert p\lvert\alpha\rangle = -i\hbar\frac{\partial}{\partial x'}\langle x'\lvert\alpha\rangle,\tag{1.7.17}$$
