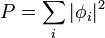$\sigma$-additivity - probability of a sum of countable number of pairly disjoint events equals a sum of probabilities of these events. (3. Axiom of Probability)
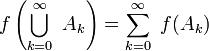
For pairly disjoint sets $A_k$
Since there are different methods of summing amplitudes in QM depending on whether outcomes of measurement are distinguishable or not (i.e. in case of identical particles and non-identical particles [Feynman vol. 3]), does it mean that "probability" in QM does not satisfy the $\sigma$-additivity which is crucial property in classical probability?
If there are several mutually exclusive, indistinguishable alternatives in which an event might occur, the probability amplitude of all these possibilities add to give the probability amplitude for that event:

When an experiment is performed to decide between the several alternatives, the same laws hold true for the corresponding probabilities and not the probability amplitudes: