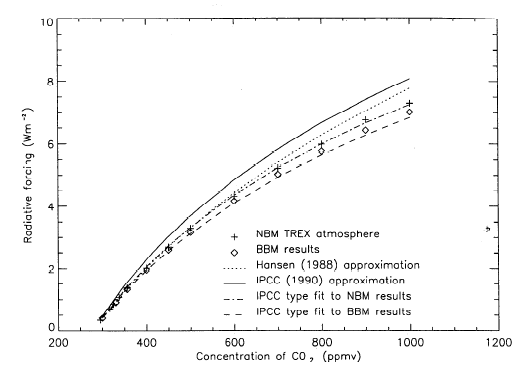
To start with, I need to write a list of assumptions that are at play, and numerous disclaimers are needed. Firstly, the IPCC scientists don't say this follows a $ln$$\ln$ function at all. They say it follows whatever their computer models says it follows. This is only a first order solution. Assumptions begin here:
$$F_{CO2} = \int_0^{\infty} \beta \Phi (1 - e^{\tau(\lambda)}) d\lambda$$$$F_{CO2} = \int_0^{\infty} \beta \Phi (1 - e^{-\tau(\lambda)}) d\lambda$$
$$(1 - e^{\tau(\lambda)}) \approx \begin{cases} 0, & \mbox{if } \tau(\lambda) < 2.3 \\ 1, & \mbox{if } \tau(\lambda) \ge 2.3 \end{cases}$$$$(1 - e^{-\tau(\lambda)}) \approx \begin{cases} 0, & \mbox{if } \tau(\lambda) < 2.3 \\ 1, & \mbox{if } \tau(\lambda) \ge 2.3 \end{cases}$$