in two dimensional electrostatics it is assumed that the whole physical system is translationally invariant in one direction. Here, the two-dimensional Laplace equation $$\Delta \phi(x,y) = \frac{\sigma(x,y)}{\epsilon_0}$$ holds in free space and solutions can be mapped on solutions if space is transformed conformally - the metric changes from $$g\rightarrow \Omega^2 g\ .$$
This approach has a lot of nice applications like the possibility to calculate the field around a complicated conductor or, in aerodynamics, the flow around an airfoil as discussed earlier.
Aubry et al. have in a number of papers (see e.g. Interaction between Plasmonic Nanoparticles Revisited with Transformation Optics (PRL) and Plasmonic Light-Harvesting Devices over the Whole Visible Spectrum (Nano Lett.)) used this technique to calculate the eigenfunctions of "crescend" structures and coupled cylinders:
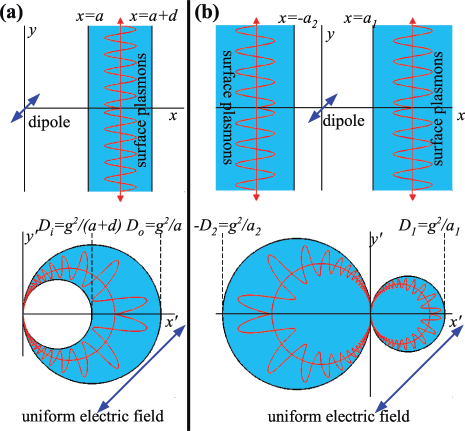 (taken from the Nano Lett.)
(taken from the Nano Lett.)
I think this is a very nice approach but I am not sure why it can be done in this way. My problem is that as far as I know usually only Neumann and Dirichlet boundary conditions are invariant under conformal transformations. In electrodynamics, however, we have that both $E_t = -d\phi(\mathbf{t})$ (tangential) and $\epsilon\cdot E_n = -\epsilon\cdot d\phi(\mathbf{n})$ (normal) are continuous at a boundary where the permittivity $\epsilon$ changes.
In the papers I could not find a discussion of this issue so I might ask here:
###Are the boundary conditions for the electrostatic potential $\phi$ invariant under conformal transformations?###
Are the boundary conditions for the electrostatic potential $\phi$ invariant under conformal transformations?
Thank you in advance
Sincerely
