I am having trouble understanding the reasoning behind the solution in this Irodov General Physics problem. TheIrodov General Physics problem is 1.6:
1.6.problem 1.6.) A ship moves along the equator to the east with velocity vo = 30 km/hour$v_0=30\;km/h$. The southeastern wind blows at an angle Θ = 60° to$\theta=60°$ to the equator with velocity v = 15 km/hour$v=15\;km/h$. Find the wind velocity v' $v'$ relative to the ship and the angle Θ'$\theta'$ between the equator and the wind wind direction in the reference frame fixed to the ship.
My calculations:
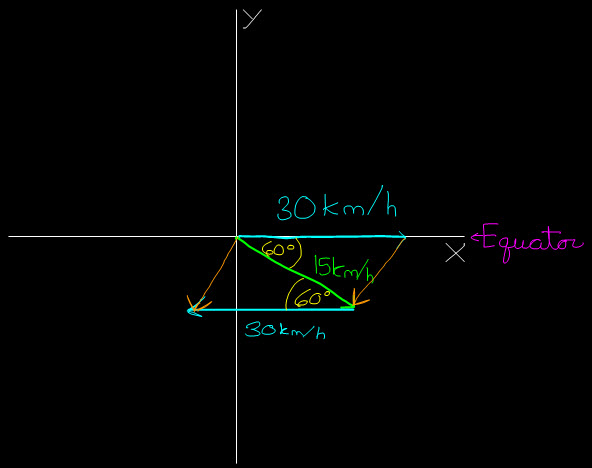
After drawing the initial diagram I consider that in order for the wind direction to be relative to the boat, I need to subtract the boat vector from the wind vector.
In my diagram I have repositioned the boat vector to be subtracted from the wind vector and using trigonometry I solve as follows:
First the cosine lawcosine law to determine the magnitude
The magnitude = $\sqrt{30^2 + 15^2 - 2\cdot 15\cdot 30\cdot \cos{60}}$
= $\sqrt{675} \approx 25.98$
Then for the angle I can use sine lawsine law
$\frac{sin(\Theta)}{30 km/h} = \frac{sin(60)}{\sqrt{675}}$$\frac{sin(\theta)}{30\frac {km}{h}} = \frac{sin(60)}{\sqrt{675}}$
$\Theta= \arcsin{30km/h \cdot\frac{sin(60)}{\sqrt{675}}}$$\theta= \arcsin{\frac{30\frac {km}{h}\cdot sin(60)}{\sqrt{675}}}$
$\Theta = 90°$$\theta = 90°$
The answer I get is therefore that the wind, in reference to the boat (as felt by the people standing on the boat), is approx 26 km/h$26\:km/h$ at an angle of 60°$60°$ South of West (in relation to the equator).
However the answer given in the solutions is always that the approximate speed of the wind is 40km/h$40\:km/h$ and the angle is approx 19°$19°$.
I have looked at the solutions and I think the best explanation is given in the following diagrams obtained from link #3:
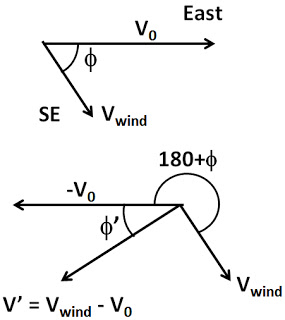

I do not understand, however, why he is using cos(180+$\Theta$)$\cos{(\pi +\theta)}$. I want to understand why my solution is incorrect and yields a different answer.
What is my mistake and what is the proper intuition for understanding this problem if I have done so incorrectly?
Solutions I have viewed:
