A wave is a perturbation in a system that propagates. The wavelength is the typical length along which a wave is coherent, which means that what happens at some position affects the wave behaviour in the vicinity if this point at distances of a few wavelengths. The reason for that is that the medium in which the wave propagates has some rigidity and the wavelength is actually determined by the rigidity of the medium.
As a consequence in diffraction, the wave field must cancel at the edge of the hole, which results in the wave feeling it. For small holes, all the wave energy feels the presence of the edges. For large holes, most of the wave field is at a distance much larger than $\lambda$, so only a small fraction of the wave is affected by the edges and the diffraction effect is not noticeable.
EDIT In answer to your comment, I will try to give a simplified explanation concerning coherence.
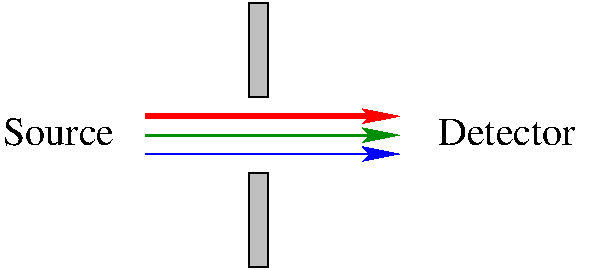
A very common picture in optics to figure out light trajectories is the following

In many situations, this is enough to understand the phenomenon (lens, mirrors, etc.). To understand what happens in diffraction, prefer the following picture

in which you notice that the rays are now beams of a certain width, depending on the color. The width you should consider is roughly the wavelength $\lambda$.
Using this picture, what happens to a light when crossing a hole. If the hole is much larger than $\lambda$, (almost) nothing happens : the beams have enough space to cross the hole
If the hole is smaller than $\lambda$, the beam will "bend" in order to keep its length and fit into the hole, as is sketched on the following picture, zoomed on the hole (I have drawn the beam as a train of stems that bend when entering the hole)
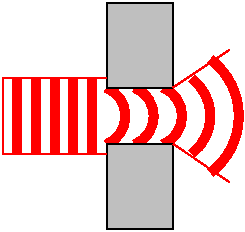
The reason for the bending is what I called coherence. It is due to the conservation of energy: if you could shrink the stems, you would lower the wavelength and thus increase the energy, which is impossible.
