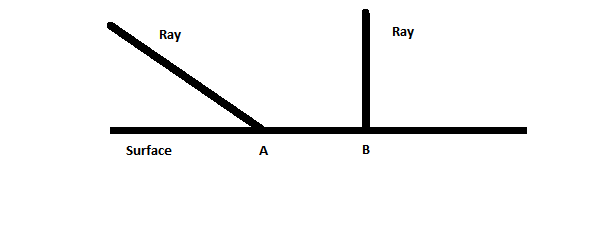
The area of the intersection of the ray with the surface at A is greater than the area of intersection at B.
To a greater area correspond more atoms to reflect the light.
EDIT add
A justification:
The equations related to graphs on Steve answer (the reflected coefficients $R_s and R_p$) are the Fresnel equations
when I read there $(n_1/n_2\cdot\sin\theta_i)^2$ I see a proportionality to the area.
The angles are in relation to the normal and sin² traces an area.
A sensivity of the equations in relation to this factor should be done to proveis apparent in the correctness of this answer. First one should convertgraphs $\cos\theta_i$ in terms($\theta_i$), because $\sin\theta_i, \sin\theta_i^2$ will 'follow' the shape of sin.($\theta_i$)
At the end II think, a priori, that my viewpoint will be validatedis justified.)
EDIT add end
the reflected rays are absent in the pic. Its not relevant.
