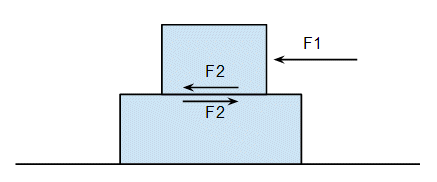
I'm guessing your FBD looked something like this, where $F_1$ is the external force you apply. I'm assuming here that the top and bottom block don't slide relative to each other, so the forces at their junction ($F_2$) are equal and opposite.
The net force on the top block is the force you apply, $F_1$, minus the frictional force the bottom block applies to the top block, $F_2$:
$$ F_{top} = F_1 - F_2 $$
Because $F_1 > F_2$ the net force $F_{top} > 0$ and the top block accelerates.
Response to comment:
If the two blocks don't slide relative to each other then their accelerations must be the same so:
$$ \frac{F_{top}}{m_{top}} = \frac{F_{bottom}}{m_{bottom}} $$
We know that $F_{top} = F_1 - F_2$ and $F_{bottom} = F_2$, so:
$$ \frac{F_1 - F_2}{m_{top}} = \frac{F_2}{m_{bottom}} $$
and a quick rearrangement gives:
$$ F_1 = F_2 \frac{m_{top} + m_{bottom}}{m_{bottom}} $$
and since $m_{top} + m_{bottom} > m_{bottom}$ this means $F_1 > F_2$.
Basically $F_2$ is only accelerating the bottom block while $F_1$ is accelerating both blocks, so $F_1$ has to be greater than $F_2$.
