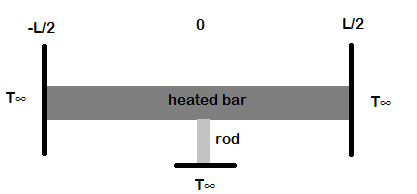
Say we have a bar centered at $x=0$, that is heated. We have the 1D Heat equation which we can solve to find a parabolic temperature profile :
$$\kappa \frac{d^2 T}{d x^2}=-\frac{Q}{L S}$$
with
$$T(L/2)=T(-L/2)=T_{\infty}$$
$T_{\infty}$ is the ambient temperature at the end of the bar. This boundary condition alone is enough to solve the equation. However, now say we want to add a very tiny rod at the center of the bar that is connected to the environment to act as a path for heat flow. $R$ is the thermal resistance of the rod. $S$ is the cross sectional area of the bar. $\kappa$ is thermal conductivity of the system. $Q$ is the heat dissipated by joule heating. The corresponding boundary condition is:
$$\frac{T(0)-T_{\infty}}{R_{\rm thermal \ rod}}=\kappa S \left(\frac{d T}{dx}_{x=0^+}-\frac{d T}{d x}_{x=0^-} \right)$$
I have fiddled around with this math for a while but cannot figure out how to implement it.
In particular I am following this paper on the T-Bridge Method for thermal conductivity.
