It was the observation of symmetries in studying nuclear physics that led to the use of group theory and multiplets. It was experimentally found that the behavior of nuclei did not depend to first order on the number of protons and neutrons, but on the number of nucleons ( either protons or neutrons). In addition it was found that a spin assignment on the nucleon of +1/2 for the proton and -1/2 for the neutron would describe economically interactions observed . This was called isotopic spin and could be organized with the group SU(2).
Then scatterings of elementary particles gave rise to the representations in multiples., because more quantum numbers were found and the symmetries found could be described with multiplets of SU(3).
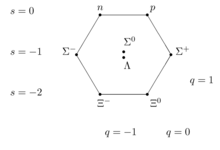
The meson octet. Particles along the same horizontal line share the same strangeness, s, while those on the same diagonals share the same charge, q.
Isospin on the x axis and strangeness quantum number on the y describe the baryon octet. The masses to first order of the isospin multiplets of the x axis are the same.

The organization into multiplets for all resonances and excitations had predictive behavior, as with the prediction of the omega- , the tip of the decouplet.
The first Omega baryon discovered was the Ω−, made of three strange quarks, in 1964.3 The discovery was a great triumph in the study of quark processes, since it was found only after its existence, mass, and decay products had been predicted by American physicist Murray Gell-Mann in 1962 and independently by Israeli physicist Yuval Ne'eman.
The symmetries observed led to the quark model of elementary particles to start with, and to the extensive use of group symmetries in the proposed theories, leading to the standard model with the SU(3)XSU(2)XU(1)$SU(3)\times SU(2)\times U(1)$ symmetries.
