This is a fundamentally pointless question because negative mass doesn't exist (or so we think!), but I'll answer anyway because the answer is so unexpected.
Suppose we take our two massive bodies:
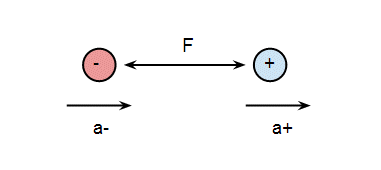
Then the gravitational force between them is repulsive because:
$$ F = \frac{G m_1 m_2}{r^2} $$
and $m_1$ and $m_2$ have different signs. But, let's calculate the resulting accelerations using Newton's second law:
$$ \vec{F} = m \vec{a} $$
With normal matter the force and acceleration are in the same direction, but if the left (red) object has a negative mass then the force and acceleration point in opposite directions. That means that even though the force between our two bodies is repulsive they both accelerate in the same direction. We get a perpetual motion machine where the two bodies will accelerate away forever. And that's as good a reason as any for supposing that negative mass doesn't exist!
