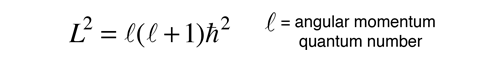Half-integer eigenvalues of orbital angular momentum

Question

Why do we exclude half-integer values of the orbital angular momentum? It's clear for me that an angular momentum operator can only have integer values or half-integer values. However, it's not clear why the orbital angular momentum only has integer eigenvalues.

Of course, when we do the experiments we confirm that a scalar wavefunction and integer spherical harmonics are enough to describe everything.

Some books, however, try to explain the exclusion of half-integer values theoretically.

• Griffiths evokes the "single-valuedness of the wavefunction" in his eq. (4.23), but he writes that that argument is not so good in a footnote. One could argue that only the probability density needs to be single-valued.

• Shankar says that the $L_z$ operator only is Hermitian when the magnetic quantum number is an integer, but his argument isn't so compelling to me.

• Gasiorowicz argues that the ladder operators don't work properly with half-integer values.

• There are some low impact papers (most of them are old) that discuss these subjects, although they are a little bit confusing.

So, basically, my question is: Does anyone have a decisive argument on why do we exclude the half-integer values from the orbital operator spectrum?

Answer 1

From $\mathbf{L}=\mathbf{Q}\times \mathbf{P}$ we have $L_z=Q_xP_y-Q_yP_x$. Then, introduce the following new operators (assuming units of $\hbar=1$): \begin{align} q_1=\frac{Q_x+P_y}{\sqrt{2}},\\ q_2=\frac{Q_x-P_y}{\sqrt{2}},\\ p_1=\frac{P_x-Q_y}{\sqrt{2}},\\ p_2=\frac{P_x+Q_y}{\sqrt{2}}. \end{align} It is immediate to check that $$ [q_1,q_2]=[p_1,p_2]=0,\quad [q_j,p_k]=i\delta_{j,k}, $$ so $q_i$ and $p_i$ are formally position and momentum operators of two different systems. It terms of these new operators $$ L_z=\frac12(p_1^2+q_1^2)-\frac12(p_2^2+q_2^2). $$ Therefore $L_z$ is nothing but the difference of two independent harmonic oscillator Hamiltonians, each having mass $M=1$ and angular frequency $\omega=1$, $L_z=H_1-H_2$, $H_i=\frac12(p_i^2+q_i^2)$. The spectrum of the harmonic oscillator Hamiltonians $H_1$ and $H_2$ is $(n_1+1/2)$ and $(n_2+1/2)$, respectively, with $n_1$ and $n_2$ positive integers. Finally, since $[H_1,H_2]=0$, the spectrum of $L_z$ is $$ (n_1+1/2)-(n_2+1/2)=n_1-n_2. $$ This is the difference of two integer numbers, so it is an integer.

Answer 2

First of all, notice that from the general theory of angular momentum, the eigevalues $m$ of $J_z$ are integer if and only if $j$ is integer because $$m = -j, -j+1,\ldots, j-1, j\:.$$

At this juncture notice that, passing from Cartesian coordinates to spherical ones, you find $$L_z= -i \partial_\phi\:.$$ From direct inspection, using this expression, you see that the eigenfunctions of $L_z$ are in $\mathbb{Z}$. Use in particular the fact that the eigenfunctions must be periodic in $[0, 2\pi]$:

$$-i\partial_\phi f(\phi) = m f(\phi)$$

implies, for some constant $C$,

$$f(\phi) = Ce^{im \phi}$$

Since $f(0)= f(2\pi)$, the only possibility is $m = 0, \pm 1, \pm 2, \ldots$ and thus $j$ is integer as well.

It is worth stressing that it is not possible to answer this question relying only on physical arguments. Physically speaking, there is no way to see the phase $-1$ associated to semi-integer $j$ for rotations of $2\pi$, since $|\psi\rangle$ and $-|\psi\rangle$ represent the same quantum state. This fact, in addition to the superposition principle leads to the superselection rule of the angular momentum.

Answer 3

In solving the Schrodinger equation for central force fields (e.g., the hydrogen atom), one generally separates variables using spherical coordinates. The result of the angular dependent equation is $$\frac{\Phi(\phi)}{\sin\theta}\frac{d}{d\theta}(\sin\theta \frac{d\Theta}{d\theta}) +\frac{\Theta(\theta)}{\sin^2\theta}\frac{d^2\Theta(\theta)}{d\phi^2}+\ell(\ell+1)\Theta(\theta)\Phi(\phi)=0$$

EDIT: The parameter $\ell$ is a partial differential equation separation parameter for the SWE. It appears in both the radial and angular parts. The solutions to the Radial equation will diverge $r \to \infty$ if $\ell$ is non-integer. Take a look at Laguerre functions and/or Arfken's Mathematical Physics section on the SWE.

If $\ell$ is an integer, the radial function will vanish as $r \to \infty$ which is required for a physically meaningful solution. This means that the angular solutions must also have integer $\ell$ and will be the associated Legendre functions: $\Theta(\theta) = P^m_{\ell}(\cos\theta) $, where $m$ is the separation constant for the $\Phi(\phi)$ solution. Ultimately, these two angular solutions form the spherical harmonics, $Y^m_{\ell}(\theta,\phi)$.

The spherical harmonics are the eigenfunctions of the square of the quantum mechanical angular momentum operator.

In summary, if $\ell$ is not an integer, there are no convergent, physically-realizable solutions to the SWE. The half-integer values do not give vanishing radial solutions.

Answer 4

1. In this answer we elaborate on Ballentine's method of finding a canonical transformation (CT) $$(q,p)\quad \longrightarrow\quad (Q,P),\tag{1}$$
   cf. NessunDorma's answer. This leads to a proof that (a finite-dimensional irrep of) the orbital angular momentum (OAM) operator has integer spin quantum number, cf. OP's title question.

2. Recall first of all that instead of position and momentum operators, we may equivalently use annihilation & creation operators $$ \begin{align} a^j~=~& \sqrt{\frac{m\omega}{2\hbar}}q^j+\frac{ip_j}{\sqrt{2m\omega\hbar}} , \cr a^{\dagger}_j~=~& \sqrt{\frac{m\omega}{2\hbar}}q^j-\frac{ip_j}{\sqrt{2m\omega\hbar}}, \cr A^j~=~& \sqrt{\frac{m\omega}{2\hbar}}Q^j+\frac{iP_j}{\sqrt{2m\omega\hbar}}, \cr A^{\dagger}_j~=~& \sqrt{\frac{m\omega}{2\hbar}}Q^j-\frac{iP_j}{\sqrt{2m\omega\hbar}} ,\cr j~\in~&\{1,2,3\}. \end{align}\tag{2}$$ Here $m\omega>0$ is some appropriate dimensionful constant whose numerical value is not important for what follows. The OAM operators are $$\begin{align} L_j~=~&\sum_{k,\ell=1}^3\epsilon_{jk\ell}q^kp_{\ell}\cr ~\stackrel{(2)}{=}~& -i\hbar\sum_{k,\ell=1}^3\epsilon_{jk\ell}a_k^{\dagger}a^{\ell} ,\cr j~\in~&\{1,2,3\}.\end{align} \tag{3}$$ The number operators are $$\begin{align} n_j~:=~&a_j^{\dagger}a^j,\quad(\longleftarrow\text{no $j$-sum})\cr N_j~:=~&A_j^{\dagger}A^j,\quad(\longleftarrow\text{no $j$-sum})\cr j~\in~&\{1,2,3\}. \end{align}\tag{4}$$

3. Consider a 1-parameter linear CT connected to the identity$^1$ $$\begin{align} Q^j~=~& q^j\cos\theta + \frac{1}{m\omega}\sum_{k=1}^2|\epsilon^{jk}| p_k\sin\theta,\cr P_j~=~& p_j\cos\theta - m\omega\sum_{k=1}^2|\epsilon_{jk}| q^k\sin\theta, \cr A^j~=~& a^j\cos\theta - i\sum_{k=1}^2|\epsilon^{jk}| a^k\sin\theta,\cr A_j^{\dagger}~=~& a_j^{\dagger}\cos\theta + i\sum_{k=1}^2|\epsilon^{jk}| a_k^{\dagger}\sin\theta,\cr j~\in~&\{1,2\}, \cr Q^3~=~& q^3,\cr P_3~=~& p_3,\cr A^3~=~& a^3, \end{align} \tag{5}$$

4. Ballentine's observation becomes $$\begin{align} N_1-N_2~\stackrel{(3)+(4)+(5)}{=}&~(n_1-n_2)\cos 2\theta\cr &+ \frac{L_3}{\hbar} \sin 2\theta.\end{align} \tag{6}$$ Of course we are mainly interested in the angle $\theta=\frac{\pi}{4}$, where the OAM $$\frac{L_3}{\hbar}~\stackrel{(6)}{=}~N_1-N_2\tag{7}$$ becomes the difference of two number operators, cf. OP's question.

5. Let $V_{\ell}$ be a finite-dimensional spin-$\ell$ irrep of the OAM Lie algebra $${\rm span}_{\mathbb{R}}(L_1,L_2,L_3)~\cong~ so(3).\tag{8}$$ We would like to prove that $\ell$ cannot be half-spin, cf. OP's question.

6. Note that $$\sum_{j=1}^3N_j~\stackrel{(4)+(5)}{=}~\sum_{j=1}^3n_j\tag{9}$$ is a Casimir operator in the sense that it commutes with $L_1$, $L_2$ & $L_3$. Therefore there exists a common set of eigenbasis $|\ell m\rangle$ such that $$\begin{align}L_3|\ell m\rangle~=~&\hbar m|\ell m\rangle,\cr \vec{L}^2|\ell m\rangle~=~&\hbar^2\ell(\ell+1)|\ell m\rangle, \cr \ell, m~\in~&\frac{1}{2}\mathbb{Z}, \cr \sum_{j=1}^3N_j|\ell m\rangle~=~&\nu |\ell m\rangle, \cr \nu~\geq~&0.\end{align}\tag{10}$$

7. Consider one such state $|\ell m\rangle$. We can act on $|\ell m\rangle$ (finitely many times) with annihilation operators $A^1$, $A^2$ & $A^3$, to reach a Fock vacuum state $|\Omega\rangle\neq 0$ with$^2$ $$A^j|\Omega\rangle~=~0,\qquad j~\in~\{1,2,3\}.\tag{11}$$ (Else there will be negative norm states by a standard argument, cf. e.g. section 6.1 in Ref. 1 or my Phys.SE answer here.)

8. In turn, this implies that $|\ell m\rangle$ is a common eigenvector for $N_1$, $N_2$ & $N_3$, with integer eigenvalues. In particular, $m$ must be an integer, cf. eqs. (7) & (10). $\Box$

9. It's interesting to note that while there are of course no genuine (as opposed to projective) half-spin irreps of the 3D rotation group $SO(3)$, the corresponding Lie algebra $so(3)$ has in principle half-spin irreps, i.e. there are no topological obstructions at the Lie algebra level. Nevertheless, as we saw above, for the OAM Lie algebra, the underlying Fock space representation of the Heisenberg algebra says otherwise! In that sense this proof is very different from a topological proof.

References:

1. L.E. Ballentine, QM modern developments, 1998; p. 170.

--

$^1$ Our notation is slightly different from Ref. 1. For starters, we prefer to use small letters before the CT (5) and capital letters after. A type 2 generating function for the CT (5) is $$\begin{align} F_2(q,P)~=~&q^3P_3+\frac{\sum_{j=1}^2q^jP_j}{\cos\theta}\cr ~+~&\frac{1}{2}\sum_{j,k=1}^2\left(m\omega|\epsilon_{jk}| q^jq^k+\frac{1}{m\omega}|\epsilon^{jk}| P_jP_k\right) \tan\theta.\end{align}\tag{12}$$

$^2$ The Fock vacuum $|\Omega\rangle$ happens to be invariant under the CT (5): $$\begin{align} a^j|\Omega\rangle ~=~&0 \cr ~\Updownarrow~& \cr A^j|\Omega\rangle ~=~&0,\cr j~\in~&\{1,2,3\}.\end{align} \tag{13}$$ Note that the Fock vacuum $|\Omega\rangle$ depends on the constant $m\omega$.

Answer 5

I had already answered this question, but my answer had a fatal flaw. This should be correct.

First, we have to make a physical demand. We demand that a rotation by $2\pi$ of spatial configuration (distinct from an internal configuration, i.e. spin) leaves physics invariant. From a study of the first homotopy group $Z_2$, we know that in general there are two possibilities for a rotation by $2\pi$ (this is usually studied in the context of topological quantization): $R(2\pi)=1$ and $R(2\pi)=-1$. For $R$ to be a physical spatial rotation, we demand $R(2\pi)=1.$ However, the rotation operator in the $x-y$ plane is $R=\exp(-i\theta L_z)$ where $L_z|\psi\rangle=m|\psi\rangle$. Thus we require $\exp(-2\pi im)=0$. This is only solved by $m\in\mathbb{Z}$, which, by the rules of ladder operators, implies $l\in\mathbb{Z}$.

Answer 6

I am going to try to answer the question, because of what is said in the comments:

It does not seem to me that the 7 answers properly answer the question! For that reason I reopened the question.

Before delving into the explanation I think it is important to note that Orbital Angular Momentum is a physical quantity that is first defined in Classical Mechanics. Its Quantum Mechanical analogue is obtained by a (reasonable) guess that the expression should have the same components, and simply translating the classical variables to quantum operators. But it did not arise directly from the symmetry principles of QM.

---

Why can't $\hat{\mathbf{L}}^2$ have half-integer quantum numbers?

We first encounter orbital angular momentum in the context of the solution to the Hydrogen atom while looking for a Complete Set of Commuting Observables to express the bound states of the system.

It is defined, in the same way as the Hamiltonian, by analogy with the classical angular momentum as:

$$\hat{\mathbf{L}}\equiv\hat{\mathbf{X}}\times\hat{\mathbf{P}}=-i\hbar\mathbf{x}\times\boldsymbol{\nabla}$$

where the last equality holds in the position and momentum representation of the state vector $\Psi$. The components of $\hat{\mathbf{L}}$ can be written as:

$$\hat L_i=-i\hbar\sum\limits_{j,k}\varepsilon_{ijk}x_j\frac{\partial}{\partial x_k}$$

where $\varepsilon_{ijk}$ is the Levi-Civita Symbol. The total orbital angular momentum, the subject of your question is then given by $\hat{\mathbf{L}}^2$,

$$\hat{\mathbf{L}}^2=\sum\limits_i\hat L_i\hat L_i=-\hbar^2\sum\limits_{i,j,k,l,m}\varepsilon_{ijk}\varepsilon_{ilm}x_j\left(\frac{\partial}{\partial x_k}\right)x_l\left(\frac{\partial}{\partial x_m}\right)$$

And skipping some steps we arrive at:

$$\hat{\mathbf{L}}^2=-\hbar^2\left(r^2\nabla^2-\frac{\partial}{\partial r}r^2\frac{\partial}{\partial r}\right)$$

Expressed in spherical coordinates $(r, \theta, \phi)$ (the total angular momentum has to be rotationally invariant, so it is expected that the operator is only a function of the $r$ coordinate).

For a reasonable$^{*}$ potential $V(\mathbf x)$ we can express $\Psi$ near the origin as a power series in the basis vectors. In particular, as $r\to 0$, only the terms with the lowest order in the series "survive":

$$\Psi(\mathbf x) \to r^{\ell}Y(\theta, \phi)$$

Here $\ell$ is the order of the lowest non-vanishing term in the power series. The $Y(\theta, \phi)$ is also a $\ell$'th order (homogeneous) polynomial in $\{\mathrm{sin}(\theta)\mathrm{cos}(\phi), \ \mathrm{sin}(\theta)\mathrm{sin}(\phi), \ \mathrm{cos}(\theta)\}$ (you may identify these as the $\{x, \ y, \ z\}$ components of a unitary vector).

Acting on this $\Psi$ with $\hat{\mathbf{L}}^2$ we get,

$$\hat{\mathbf{L}}^2\Psi \to \hbar^2\ell(\ell+1)\Psi$$

Now, if $\Psi$ is an eigenfunction of $\hat{\mathbf{L}}^2$ then the eigenvalue should be the same for any and every point, so we conclude that the eigenvalue of such a function must be $\hbar^2\ell(\ell+1)$.

This is as far as we can go with angular momentum in the position-momentum representation. As we saw above all (reasonable) wavefunctions, including the eigenfunctions of $\hat{\mathbf{L}}^2$ can be expressed as $\propto r^\ell$ for small $r$. And there are no fractional power series$^{**}$, it follows that the orbital angular momentum only has (positive) integer quantum numbers.

So, to respond your question,

Does anyone have a decisive argument on why do we exclude the half-integer values from the orbital operator spectrum?

Because the orbital angular momentum was chosen to match our classical definition of angular momenta. This leads to the above expression of $\hat{\mathbf{L}}^2$ with its corresponding limitations.

At least that's how I interpret it.

---

How do half-integer angular momenta appear?

As we have seen, the position-momentum representation only gives us integer angular momenta, for more general angular momenta we need to rely on symmetry principles.

Symmetries are transformations that leave the equations of motion, and the physical content of the mathematical representations invariant. In classical mechanics the symmetries are encoded in the Galilean group: space rotations, translations in space and time and galilean transformations should not change the outcome of an experiment (if I perform an experiment under the same conditions it shouldn't matter if I do it today or tomorrow, or if I do it in Hansford or Livingston).

In quantum mechanics, all the transformations acting on the state vectors should be Unitary, in order to preserve the inner product (and therefore the probabilities). That is,

$$\langle\hat{U}\Psi\vert\hat{U}\Phi\rangle = \langle\Psi\vert\Phi\rangle$$

It follows that $\hat{U}^{\dagger}\hat{U}=\hat{1}$. For infinitesimal transformations (for example, rotation by an infinitesimal angle), the Unitary operators take the following form:

$$\hat U = \hat{1}+i\epsilon \hat T+\cdots$$

where $\epsilon$ is the "infinitesimal" (arbitrarily small) number. Here, the operator $\hat T$ is hermitian. In this context $\hat T$ is called the generator of the transformation (you could repeat the infinitesimal transformation an infinite number of times to get a finite transformation and the only information you need to do that is $\hat T$). You can use this to show that the momentum operator is the generator of space translations, and the Hamiltonian is the generator of time translations.

If you know what form $\epsilon$ takes (it is related to the transformation in question) you can exploit the unitary condition $\hat{U}^{\dagger}\hat{U}=\hat{1}$ to arrive at the commutator relations for the operator $\hat T$. The particular case of the angular momentum ($\hat T =\hat{\mathbf{J}}$) is shown in chapter 4, section 1 of Weinberg's Lectures on Quantum Mechanics. I will omit the details of the derivation, but what you arrive at is:

$$\left[\hat J_i, \hat J_j\right]=i\hbar\sum\limits_k\varepsilon_{ijk}\hat J_k$$

As you can check, $\hat{\mathbf{L}}$ also satisfies the commutation relations$^{***}$. In general we can express $\hat{\mathbf{J}}$ as,

$$\hat{\mathbf{J}}=\hat{\mathbf{L}}+\hat{\mathbf{S}}$$

We have already seen that $\hat{\mathbf{L}}$ has just integer angular momenta, so the only place for the half-integers is $\hat{\mathbf{S}}$. Additionally, with this you can check that $\left[\hat S_i, \hat L_j\right]=0$, and also, $$\left[\hat S_i, \hat X_j\right]=\left[\hat S_i, \hat P_j\right]=0$$

What this last equation means is that $\hat{\mathbf{S}}$ is independent of $\hat{\mathbf{X}}$ and $\hat{\mathbf{P}}$, and this is why we can't see the spin in the position-momentum representation.

---

In the symmetry part I omitted a lot of things not to make the answer too extensive. The source for most of my answer is chapters 2,3 and 4 of:

Weinberg, S. (2015). Lectures on Quantum Mechanics. Cambridge: Cambridge University Press.

---

$^{*}$ In the time independent Schrödinger equation we have $\frac{\hbar^2}{2m}\nabla^2\Psi=(V-E)\Psi$, $E$ is finite but $V$ may diverge (like in the Hydrogen atom), but if the divergence is not very singular then the first derivatives of $\Psi$ will be at least finite and $\Psi$ itself will be continuous everywhere (and differentiable everywhere except possibly at the singular point)

$^{**}$ For instance $r^{\frac{1}{2}}$ doesn't even have a series expansion at $r=0$ whereas higher order half-integers of the form $a+\frac{1}{2}$, for $a\in\mathbb N$, have their first $a$ derivatives at $r=0$ equal to zero (so it is a trivial expansion) and their $(a+1)$'th derivative diverge (so the expansion is undefined).

$^{***}$ There is an important difference. For any vector operator $\hat{\mathbf{V}}$, the commutation relation $\left[\hat J_i, \hat V_j\right]=i\hbar\sum\limits_k\varepsilon_{ijk}\hat V_k$ holds, but this is not true if we change $\hat{\mathbf{J}}$ for $\hat{\mathbf{L}}$.

Answer 7

There is a simple reason why orbital angular quantum numbers must be integers. Any wave function may be expressed as a column vector of components $f_i({\bf r})$. For example, a 3-D vector field has three components $(f_x({\bf r}), f_y({\bf r}), f_z({\bf r}))$. A 2-spinor has components $(f_{up}({\bf r}), f_{down}({\bf r}))$. Rotation changes the wave function via the spin matrices that mix different components, and via rotation of the functional argument (the position $\bf{r}$). Since a $2 \pi$ rotation returns the position to its original location, the Fourier decomposition of $f_i(r, \phi,z)$ can only depend on integer multiples of $\phi$. Since $L_z = -\text{i} \partial_\phi$ in these coordinates, orbital quantum numbers are integers.

Another way to see this is that at the origin (${\bf r} = 0$), behavior of the wave function under $2 \pi$ rotation is determined only by the eigenvalues ($m_s$) of the spin matrices since ${\bf L} = {\bf r} \times {\bf p} = 0$ at the origin. Rotation of $2 \pi$ around a neighboring point must have the same effect on the wave function, so including position-dependence in the amplitudes $f_i({\bf r})$ does not change the effect of a $2 \pi$ rotation. This again implies that the orbital quantum numbers ($m_L$) must be integers, and not half-integers.

Answer 8

So, basically, my question is: Does anyone have a decisive argument on why do we exclude the half-integer values from the orbital operator spectrum?

Lets go back to basics.

To start with the eigenvalues of angular momentum are not integer . Not even an integer multiple , as a square root gets in the way.

This was discovered while solving the Schrodinger equation, and the great success of being able to fit the atomic spectra with the quantum numbers given by the solution of the wave equation gives validity to the definition. It so happens that $l$ is an integer. So fitting the data is responsible for l being integer in the formula above , as the equation that does so comes out with the above formula for angular momenta. If the data would be fitted with half integer $l$ that is what we would have been stuck with.

Half integer spin quantum numbers also are defined by the data, clearly, in particle physics interactions. Conservation of angular momentum is an axiomatic statement in classical physics ( because that is what measurements tell us), in elementary particle interactions, half integer spins were necessarily axiomatically assigned to electrons, protons, neutrons and neutrinos so that the interactions would conserve angular momentum. Thus angular momentum conservation is preserved as an axiomatic statement in quantum interactions too,

So it is the data that define the angular momentum . The obvious fact that one can define complicated field theoretical mathematical forms with angular momentum operators should not obscure the fact that that is what the data tells us.

Answer 9

We define the basic angular momentum state by the action of the $z$-component angular momentum operator $L_z$ and the squared total angular momentum operator $L^2=\sum_{i=1}^3L_iL_i$: $$L_z|l,m\rangle=m|l,m\rangle\quad\text{and}\quad L^2|l,m\rangle=l(l+1)|l,m\rangle$$ As you probably know, we define raising and lowering operators $$L_\pm=L_x\pm iL_y$$ that act on states like $$L_\pm|l,m\rangle=\sqrt{l(l+1)-m(m\pm1)}|l,m\pm1\rangle$$ Now we prove that only integer eigenvalues make sense by contradiction. Say we measure angular momentum along the $z$-axis. We say that the particle is at rest if $m=0$. By using the raising and lowering operators, we get the usual $-l,-l+1,\dots,l-1,l$ spectrum. Suppose, however, we perform the measurement $$L_z|l,m\rangle=\tfrac{1}{2}|l,m\rangle$$ This does not make sense, though. It would appear that, by going in integer steps, we cannot make the particle be at rest. This is intrinsic angular momentum, namely spin. If we want the particle to be able to have an $m=0$ state along the $z$-axis, orbital angular momentum must be integer quantized.

Answer 10

We exclude it because doing so agrees with experiment. But through a lot of song and dance you can argue that the angular momentum operator that enters the Hamiltonian is a vector after all, and vectors transform under integer representations of SU(2) rather than half integers.

As for single valuedness of the wave function, well, if $\psi$ jumped discontinuously at some angle, then the angular momentum operator would be infinite at that point. Thus $psi$ wouldn't truly be an eigenfunctions.

Answer 11

Consider the simplest case, the movement in a spherically symmetrical potential. The Hamiltonian becomes,

$$-\frac {\hbar^2}{2m}\left[\frac {1}{r^2} \frac {∂}{∂r} \left(\frac {r^2∂}{∂r}\right) + \frac {1}{r^2\sin\theta} \frac {∂}{∂\theta} \left(\sin\theta \frac {∂}{∂\theta}\right) + \frac {1} {r^2\sin^2\theta}\frac {∂^2}{∂\phi^2}\right] + V(r)$$

There is nothing in this Hamiltonian to hint that at the rotation of the solution by $2\pi$ the wave-function should change sign.