How fast does an ice cube melt in a microwave?

Question

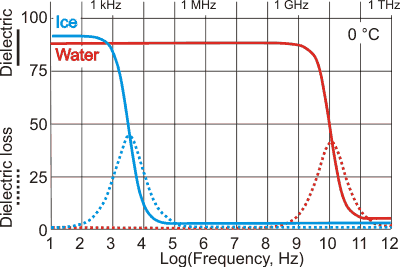
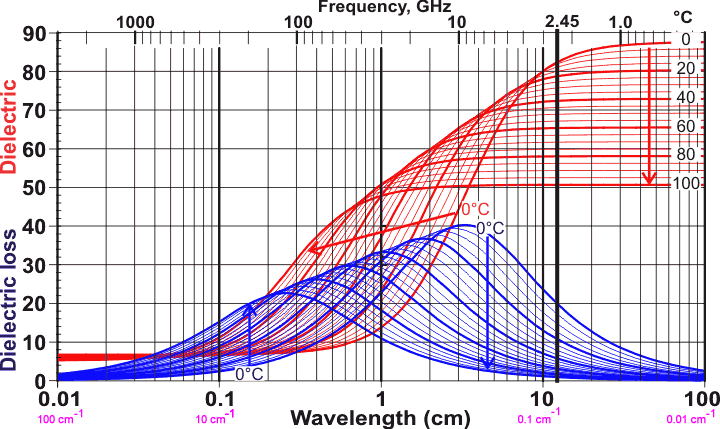
I have noticed that when I microwave an ice cube it appears to melt more slowly than I would expect. For example, an equal volume of water starting at 0 deg C would probably be at boiling point before an ice cube that was at -15 deg C had melted. I realize there is enthalpy of fusion to take into account in the melting process but I believe there is more to it than that.

As I understand it a microwave oven works by exciting the water molecules in whatever is being cooked and if memory serves the frequency used is one that causes rotation of the molecule. Since the ice cube is solid I'm assuming the molecules aren't free to rotate and therefore the microwaves have a much reduced effect. In fact I'm wondering if a perfect single crystal of water would respond at all to being microwaved. Does this sound right?

I've been trying to rack my brain for a way of testing this theory but I can't think of a way of getting an perfectly dry ice cube into a microwave to see if anything happens. Even a tiny amount of surface water, caused from interaction with a warm atmosphere, would encourage melting.

Answer 1

I stumbled on this question rather late - and when the link to the image in @Georg's answer was no longer working I started a little digging of my own. I came upon the following plot (at https://water.lsbu.ac.uk/water/microwave_water.html) which explains this very well:

It shows unambiguously that water has a strong absorption peak in the "low GHz" range (right around the microwave) while the absorption peak for solid ice happens at a much lower frequency - about 6 orders of magnitude lower.

The article goes on to explain this by stating that the dipole in the water molecule attempts to align with the changing electric field; when the phase difference of this alignment is at 90 degrees (resonance) the heat transfer is maximized. For liquid water you are near resonance - for ice, you are far away. Quoting from the page (I put key phrases in bold):

The water dipole attempts to continuously reorient in electromagnetic radiation's oscillating electric field (see external applet). Dependent on the frequency the dipole may move in time to the field, lag behind it or remain apparently unaffected. When the dipole lags behind the field then interactions between the dipole and the field leads to an energy loss by heating, the extent of which is dependent on the phase difference of these fields; heating being maximal twice each cycle. The ease of the movement depends on the viscosity and the mobility of the electron clouds. In water these, in turn, depend on the strength and extent of the hydrogen bonded network. In free liquid water this movement occurs at GHz frequencies (microwaves) whereas in more restricted 'bound' water it occurs at MHz frequencies (short radiowaves) and in ice at kHz frequencies (long radio waves).

Incidentally - and I admit, to my surprise - it seems that the resonance peak for liquid water shifts quite a bit with temperature; see this graph from the same source (I don't quite understand what the units are… but the general shape and direction with temperature are evident; note the 2.45 GHz line which corresponds to the typical frequency of the home microwave oven):

At 2.45 GHz, the dielectric absorption decreases as temperature goes up. This suggests that cold water heats more rapidly than hot water, but I haven't attempted to measure this myself. Might be a fun follow-up for somebody. I think that "microwave physics" is an underused topic for school science fair experiments…

Answer 2

The unusual thing is the really high absorption of microwaves by bulk water, whereas the ice behaves more normal like most solids and liquids. In liquid water we have an effect of relaxation of orientational polarisation. The polarisation is achieved not by rotation (not possible in liquid water) but by shift of hydrogen atoms along the hydrogen bonds. This is a kind of Kohlrausch conduction mechanism. This process is extremely fast, so polarisation of water is one of the fastest processes in liquids. There is debate, whether tunneling plays a role to enhance the shift of the protons. The same mechanism is responsible for the extraordinary (about tenfold) mobilities of H+ Ions in water.

Here is a plot of Water spectrum in microwave domain. Note the incredible absorption maximum with k about 3 !

http://books.google.com/books?id=bj1EnQPB0CMC&pg=PA184#v=onepage&q&f=false

Answer 3

This is a nice application of $W=F\Delta x$ for mechanical work, or its rotational analogue $W=\tau\Delta\theta$. Molecules in a liquid are free to rotate, so $\Delta\theta$ can be large. If you think of the lattice in an ice crystal as being built out of individual water molecules, then $\Delta\theta$ is limited by the ability of the lattice to deform, and you get a much smaller amount of work done by the electric field.

If you try melting a stick of butter in the microwave, you'll see that it doesn't melt evenly. The melting starts in a certain spot, and then the spot spreads. The idea here is that once you get even a tiny bit of liquid, that liquid becomes efficient at absorbing energy, so the process snowballs. For this reason, I don't think you can test the theory by trying to use ice that has been thoroughly dried. As soon as the tiniest amount of liquid forms, it starts to grow.

Some possible experimental tests:

The explanation is independent of the detailed nature of the substance, so it should be true for all substances that the solid heats more slowly than the liquid. This seems to be verified by the fact that butter acts the same way as water.

An insulating liquid without any electric dipole moment should heat more slowly than one like water that has a dipole moment. I don't know of a safe, easy example, though.

[EDIT] Reading a little more on the web, it turns out that there are four qualitatively different effects by which microwaves can heat matter:

1. dielectric heating -- This is the effect I described above.

2. ionic conductivity

3. electronic conductivity

4. hysteresis

In most foods, 1 and 2 are of approximately equal strength. 3 can occur in soot particles formed when food burns. In pure water and ice, only 1 is significant.